Technical note: A new online tool for δ¹⁸O–temperature conversions

The stable-oxygen-isotopic composition of marine carbonates (δ¹⁸O_c) is one of the oldest and most widely used paleothermometers. However, interpretation of these data is complicated by the necessity of knowing the δ¹⁸O of the source seawater (δ¹⁸O_w) from which CaCO₃ is precipitated. The effect of local hydrography (the “salinity effect”) is particularly difficult to correct for and may lead to errors of >10 ^∘C in sea-surface temperatures if neglected. A variety of methods for calculating δ¹⁸O_w have been developed in the literature, but not all are readily accessible to workers. Likewise, temperature estimates are sensitive to a range of other calibration choices (such as calibration species and the inclusion or exclusion of carbonate ion effects), which can require significant effort to intercompare. We present an online tool for δ¹⁸O–temperature conversions which provides convenient access to a wide range of calibrations and methods from the literature. Our tool provides a convenient way for workers to examine the effects of alternate calibration and correction procedures on their δ¹⁸O-based temperature estimates.

1 Motivation

The stable-oxygen-isotopic composition of carbonates (δ¹⁸O_c) is one of the oldest and most widely used paleothermometers and undergirds a wide variety of paleoceanographic research (for recent reviews, see Pearson, 2012, and Sharp, 2017). Converting δ¹⁸O_c to temperature is typically done using an empirical calibration in either a linear form, such as

$$\begin{array}{}\text{(1)}& T=\mathrm{16.5}-\mathrm{4.80}\left({\mathit{\delta }}^{\mathrm{18}}{\mathrm{O}}_{\mathrm{c}}-{\mathit{\delta }}^{\mathrm{18}}{\mathrm{O}}_{\mathrm{w}}-\mathrm{0.27}\right),\end{array}$$

(Bemis et al., 1998), or in a quadratic form, such as

$$\begin{array}{}\text{(2)}& \begin{array}{rl}T& =\mathrm{16.0}-\mathrm{5.17}\left({\mathit{\delta }}^{\mathrm{18}}{\mathrm{O}}_{\mathrm{c}}-{\mathit{\delta }}^{\mathrm{18}}{\mathrm{O}}_{\mathrm{w}}-\mathrm{0.20}\right)\\ & +\mathrm{0.09}{\left({\mathit{\delta }}^{\mathrm{18}}{\mathrm{O}}_{\mathrm{c}}-{\mathit{\delta }}^{\mathrm{18}}{\mathrm{O}}_{\mathrm{w}}-\mathrm{0.20}\right)}^{\mathrm{2}},\end{array}\end{array}$$

(McCrea, 1950, as reformulated by Bemis et al., 1998), where T is temperature (in ^∘C), δ¹⁸O_c is the oxygen isotope composition of the carbonate (as ‰ VPDB, or parts per thousand relative to the Vienna Pee Dee Belemnite standard), and δ¹⁸O_w is the oxygen isotope composition of the water in which the carbonate was precipitated (as ‰ VSMOW, or parts per thousand relative to the Vienna Standard Mean Ocean Water standard). Much of the complexity of using δ¹⁸O as a paleothermometer arises from the need to know δ¹⁸O_w, which may vary both globally as a function of ice volume and locally at the sea surface as a function of regional hydrography (Rohling, 2013). Global variation can be estimated using independent records of sea level, so the global record of deep-water δ¹⁸O-based temperatures has been relatively well established (Zachos et al., 2001; Cramer et al., 2009; Westerhold et al., 2020; Rohling et al., 2021; etc.). However, local variations in surface δ¹⁸O_w are more difficult to predict, rendering sea-surface temperature (SST) estimates from δ¹⁸O less reliable than deep-water temperature estimates. To address this, a variety of methods have been developed in the literature to estimate surface δ¹⁸O_w.

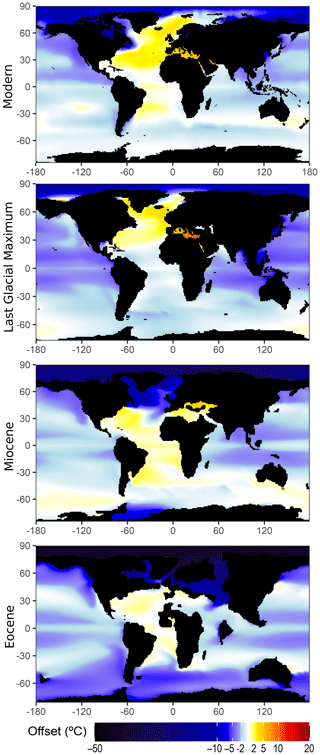
Figure 1 Effect of estimating SST using measured/modeled local δ¹⁸O_w rather than the latitude-based approximation of Zachos et al. (1994, Eq. 1 therein). Modern: comparison with mean annual δ¹⁸O_w<50 m depth (after LeGrande and Schmidt, 2006). Last Glacial Maximum (LGM): comparison with inferred annual surface δ¹⁸O_w at the LGM (Tierney et al., 2020). Miocene: comparison with CESMv1.2_CAM5 model run at 400 ppm CO₂ with Miocene paleogeography (Gaskell et al., 2022). Eocene: comparison with CESM_1.2_CAM5 model run at 6 times the preindustrial CO₂ with Eocene paleogeography (Zhu et al., 2020). Temperatures are calculated assuming a slope of 4.80 ^∘C ‰⁻¹ (Bemis et al., 1998).

Since modern surface δ¹⁸O_w broadly covaries with latitude, a common approach has been to apply the modern latitudinal variation to a sample's paleolatitude (typically using the relationship fit from Southern Ocean data in Eq. 1 of Zachos et al., 1994, or more recently the updated method of Hollis et al., 2019). However, this approach performs particularly poorly in the North Atlantic and other high northern latitudes, where local δ¹⁸O_w can deviate significantly from the latitudinal mean (Fig. 1; Zachos et al., 1994; Gaskell et al., 2022; see also generally Tindall et al., 2010). It also assumes that the latitudinal gradient in δ¹⁸O_w has not changed through time, which is contradicted by modeling. In warmer climates with an altered hydrological cycle, models predict that regional salinity contrasts should change due to alterations in the local ratio of evaporation to precipitation (Richter and Xie, 2010; Singh et al., 2016), with an analogous effect on δ¹⁸O_w (Zhou et al., 2008; Tindall et al., 2010; Roberts et al., 2011; Zhu et al., 2020). In particularly extreme cases such as the Eocene, the theoretical difference between modern latitude-derived δ¹⁸O_w (after Zachos et al., 1994, Eq. 1 therein) and modeled local δ¹⁸O_w at 6 times the preindustrial pCO₂ (Zhu et al., 2020) yields a mean temperature error of 5 ^∘C in the Southern Ocean (60–90^∘ S) or an astonishing mean temperature error of 41 ^∘C above the Arctic Circle (66.5–90^∘ N; Fig. 1).

An alternative approach is to obtain δ¹⁸O_w more or less directly from isotope-enabled climate models (Zhou et al., 2008; Roberts et al., 2011; Gaskell et al., 2022). Several approaches have been adopted: drawing local δ¹⁸O_w directly from model output (Roberts et al., 2011); using modeled zonal mean δ¹⁸O_w for a particular paleolatitude (Zhou et al., 2008); using models as input to fit a generalized equation for predicting δ¹⁸O_w from latitude and bottom-water temperature (Gaskell et al., 2022 Eq. S9); or, recently, a generalized method which uses bottom-water temperature to interpolate local δ¹⁸O_w between models run at different pCO₂ (Gaskell et al., 2022). While some authors have avoided these approaches altogether due to the uncertainty in modeled δ¹⁸O_w (e.g., Hollis et al., 2012) or the possibility of introducing circularity into data–model comparisons (e.g., Hollis et al., 2019), model-derived δ¹⁸O_w clearly captures information lost by simpler approaches and is therefore appropriate for some use cases (Roberts et al., 2011).

Here, a new online tool for δ¹⁸O temperature conversion is presented which automates a range of methods for δ¹⁸O_w reconstruction and correction from the literature, improving the accessibility of advanced methods to workers generating δ¹⁸O_c data.

2 Description

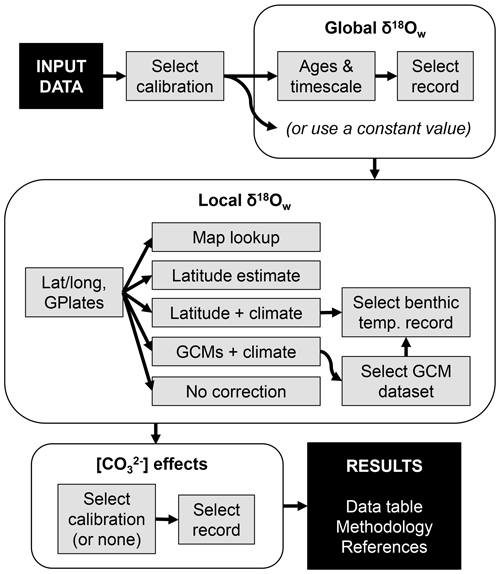
We present a new online tool for performing δ¹⁸O_c–temperature conversions which automates a range of methods from the literature. This tool is available at https://research.peabody.yale.edu/d180/ (last access: 7 June 2023). The general workflow for using the tool is summarized in Fig. 2; details on the methodology and reasoning behind each option are given below.

Figure 2 General workflow for using the tool. (Each box may reflect multiple sub-options.)

2.1 δ¹⁸O_c–temperature calibration

After manually entering or uploading a data sheet of δ¹⁸O_c measurements in .csv format, users may select from 1 of 59 different calibrations from the literature (Bemis et al., 1998; Böhm et al., 2000; Bouvier-Soumagnac and Duplessy, 1985; Duplessy et al., 2002; Epstein et al., 1953; Erez and Luz, 1983; Farmer et al., 2007; Geffen, 2012; Godiksen et al., 2010; Grossman and Ku, 1986; Høie et al., 2004; Juillet-Leclerc and Schmidt, 2001; Kim and O'Neil, 1997; Kim et al., 2007; Lynch-Stieglitz et al., 1999; Malevich et al., 2019; Marchitto et al., 2014; McCrea, 1950; Mulitza et al., 2003; O'Neil et al., 1969; Patterson et al., 1993; Reynaud-Vaganay et al., 1999; Rosenheim et al., 2009; Shackleton, 1974; Storm-Suke et al., 2007; Thorrold et al., 1997; Tremaine et al., 2011; White et al., 1999; Willmes et al., 2019). All data are expressed with δ¹⁸O_c in units of ‰ VPDB and δ¹⁸O_w in units of ‰ VSMOW, with any standard interconversions expected by the chosen calibration performed automatically. Standard interconversion is notably inconsistent in the literature, with many paleoceanographic papers employing the relationship ${\mathit{\delta }}^{\mathrm{18}}{\mathrm{O}}_{\mathrm{VPDB}}={\mathit{\delta }}^{\mathrm{18}}{\mathrm{O}}_{\mathrm{VSMOW}}-\mathrm{0.27}$ ‰ (Hut, 1987), while many geochemical papers employ the incompatible relationship ${\mathit{\delta }}^{\mathrm{18}}{\mathrm{O}}_{\mathrm{VPDB}}=\mathrm{0.97001}\times {\mathit{\delta }}^{\mathrm{18}}{\mathrm{O}}_{\mathrm{VSMOW}}-\mathrm{29.99}$ ‰  (Brand et al., 2014). The former is actually the isotopic offset between the related VPDB-CO₂ and VSMOW-CO₂ scales, but the difference is unimportant so long as all data are treated in the manner the calibration expects, as our tool ensures. A full list of included calibrations and standard conversions is given in Tables 1–3.

Table 1 Linear and quadratic δ¹⁸O: temperature calibrations of the form $T=a+b{\mathrm{\Delta }}^{\mathrm{18}}{\mathrm{O}}_{\mathrm{c}}+c({\mathrm{\Delta }}^{\mathrm{18}}{\mathrm{O}}_{\mathrm{c}}{)}^{\mathrm{2}}$, where ${\mathrm{\Delta }}^{\mathrm{18}}{\mathrm{O}}_{\mathrm{c}}={\mathit{\delta }}^{\mathrm{18}}{\mathrm{O}}_{\mathrm{c}}-{\mathit{\delta }}^{\mathrm{18}}{\mathrm{O}}_{\mathrm{w}}$, with the given VSMOW conversion factor first added to δ¹⁸O_w to convert VSMOW into the format expected by the calibration.

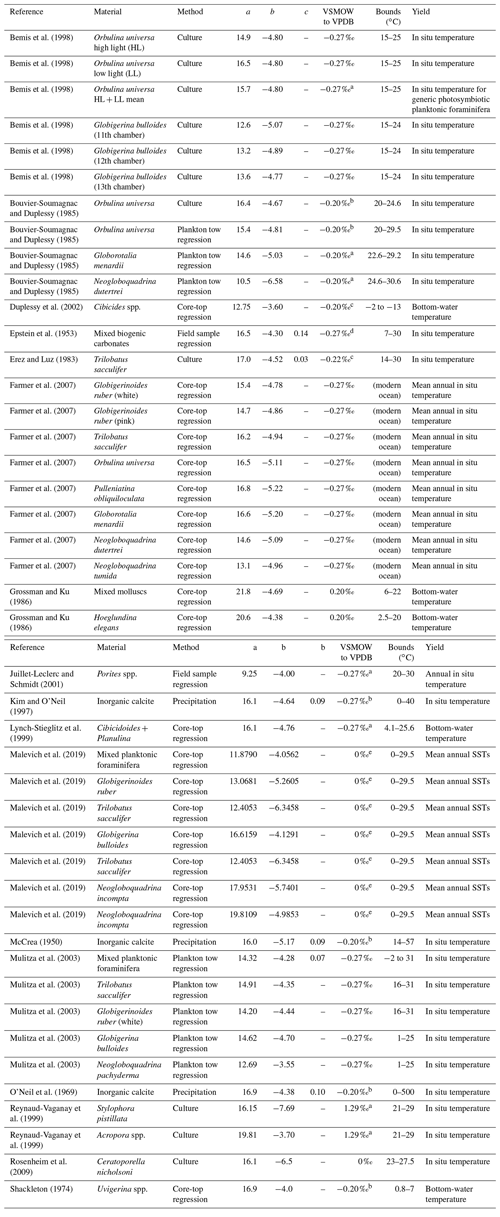
^a Rearranged by this work; ^b reformulated by Bemis et al. (1998); ^c reformulated by Mulitza et al. (2003); ^d reformulated by Bemis et al. (1998), with VSMOW correction after Grossman (2012); ^e reformulated in this work by extracting the linear coefficients from the Bayesian posterior values.

Table 2 Logarithmic δ¹⁸O: temperature calibrations of the form $\mathrm{1000}\ln \mathit{\alpha }=a\left({\mathrm{10}}^{\mathrm{3}}T{K}^{-\mathrm{1}}\right)-b$, where TK is temperature (in kelvin), and α is the fractionation factor $\mathit{\alpha }=\frac{{\mathit{\delta }}^{\mathrm{18}}{\mathrm{O}}_{\mathrm{c}}+\mathrm{1000}}{{\mathit{\delta }}^{\mathrm{18}}{\mathrm{O}}_{\mathrm{w}}+\mathrm{100}}$. The temperature solution for this form (in ^∘C) is $T=\frac{a\times {\mathrm{10}}^{\mathrm{3}}}{\mathrm{1000}\ln \left(\frac{{\mathit{\delta }}^{\mathrm{18}}{\mathrm{O}}_{\mathrm{c}}+\mathrm{1000}}{{\mathit{\delta }}^{\mathrm{18}}{\mathrm{O}}_{\mathrm{w}}+\mathrm{1000}}\right)+b}-\mathrm{273.15}$, where the relationship δ¹⁸O_VPDB=0.97001 δ¹⁸O_VSMOW−29.99 (Brand et al., 2014) is first applied to convert δ¹⁸O_c from VPDB to VSMOW or to convert δ¹⁸O_w from VSMOW to VPDB, as required by the calibration. (The value requiring conversion is indicated in the “Convert which” column.)

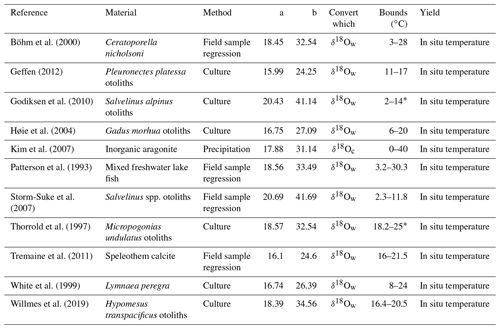
^* Reformulated by Willmes et al. (2019).

Table 3 δ¹⁸O: temperature calibrations in other forms. ${\mathrm{\Delta }}^{\mathrm{18}}{\mathrm{O}}_{\mathrm{c}}={\mathit{\delta }}^{\mathrm{18}}{\mathrm{O}}_{\mathrm{c}}-{\mathit{\delta }}^{\mathrm{18}}{\mathrm{O}}_{\mathrm{w}}$; the VSMOW–VPDB conversion is included in the equations below, with no further conversion required.

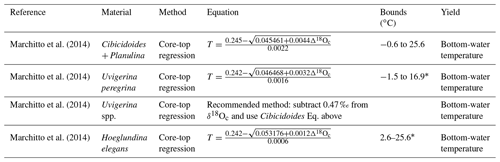
^* Rearranged by this work.

Where applicable, we use the standardized reformulations of Bemis et al. (1998) and Willmes et al. (2019) or exact algebraic rearrangements of the original equations. For the bayfox core-top calibrations of Malevich et al. (2019), the standard bayfox tool re-fits the calibration coefficients with every run. Since this is computationally expensive, we instead use the linear calibration coefficients fit by runs of the R package bayfoxr 0.0.1 directly in linear functions of the form of Eq. (1) (see Table 1). These yield results equivalent to the full fitting process within numerical error (mean residual = ±0.02 ^∘C, identical to the mean scatter between replicates of the full bayfox fit).

2.2 Global δ¹⁸O_w estimation

Users may specify global δ¹⁸O_w manually or choose to draw δ¹⁸O_w by sample age from 12 different time series of global δ¹⁸O_w from the literature (from Cramer et al., 2011; Henkes et al., 2018; Meckler et al., 2022; Miller et al., 2020; Modestou et al., 2020; Rohling et al., 2021; and Veizer and Prokoph, 2015). These records are typically constructed by assuming that the benthic δ¹⁸O record reflects a combination of temperature and ice volume and then subtracting out an independent record of temperature (e.g., using $\mathrm{Mg}/\mathrm{Ca}$-based bottom-water temperatures; Cramer et al., 2011) or ice volume (e.g., using a multi-proxy sea level reconstruction; Rohling et al., 2021) to determine the residual δ¹⁸O_w. Which global δ¹⁸O_w record is most realistic remains a contentious topic in the literature, with sea-level and $\mathrm{Mg}/\mathrm{Ca}$-based records (e.g., Cramer et al., 2011; Rohling et al., 2021) predicting up to ∼1 ‰ lower δ¹⁸O_w for much of the Cenozoic than records based on clumped isotope paleothermometry (Meckler et al., 2022; see also Agterhuis et al., 2022). We provide both classes of record here for comparison by the user.

Records are mapped to the user data's ages by linear interpolation. The Δ47-based δ¹⁸O_w records of Meckler et al. (2022) included in our tool were generated by interpolating the authors' original results to 0.1 Myr resolution using the Monte Carlo LOESS method and parameters described in the original publication (Meckler et al., 2022).

All built-in δ¹⁸O_w and temperature records are internally converted to four different timescales, so the user can select the timescale consistent with their data: GTS2004 (Gradstein et al., 2005), GTS2012 (Gradstein et al., 2012), GTS2016 (Ogg et al., 2016), and GTS2020 (Gradstein et al., 2020). These timescale conversions are performed by linear interpolation between magnetochron boundaries; dataset files can be found on the project GitHub.

2.3 Local δ¹⁸O_w estimation

The user may select a method for estimating local δ¹⁸O_w. These are as follows: performing no local correction, using modern δ¹⁸O_w from each sample's location and a specified depth (after LeGrande and Schmidt, 2006), using reconstructed Late Holocene or Last Glacial Maximum surface δ¹⁸O_w from each sample's location (model output from Tierney et al., 2020), using δ¹⁸O_w estimated from latitude alone (after Eq. 1 in Zachos et al., 1994, or the method of Hollis et al., 2019), using δ¹⁸O_w estimated from latitude and bottom-water temperature (after Gaskell et al., 2022 Eq. S9), or using δ¹⁸O_w estimated from isotope-enabled climate models (GCMs; after the method of Gaskell et al., 2022, presently provided using the datasets of Miocene and Eocene paleogeography used in that publication).

For methods which draw from an existing dataset of δ¹⁸O_w, the user may specify a number of degrees latitude/longitude or great-circle radius to average over in order to capture a regional mean when the exact paleocoordinates or local hydrography may not be known. To help determine site locations at the time of deposition, an option is also provided to automatically perform paleocoordinate rotations using the GPlates Web Service (Müller et al., 2018). Ages passed to GPlates are rounded to the nearest 100 ka to reduce the number of calls to the web service.

Our tool does not currently implement any automated consideration of seasonal variation in local δ¹⁸O_w, as this is generally treated as negligible by standard methodologies or implicitly baked into the calibration by calibrating against mean annual temperatures and δ¹⁸O_w values (e.g., Malevich et al., 2019).

2.4 Carbonate chemistry effects

Because δ¹⁸O_c is known to vary with aqueous carbonate chemistry (the “carbonate ion effect”; Spero et al., 1997; Bijma et al., 1999; Ziveri et al., 2012), users may also specify a carbonate ion correction factor. This is performed by adjusting δ¹⁸O_c with the linear relationship

$$\begin{array}{}\text{(3)}& {\mathit{\delta }}^{\mathrm{18}}{\mathrm{O}}_{\mathrm{c}}{}^{\prime }={\mathit{\delta }}^{\mathrm{18}}{\mathrm{O}}_{\mathrm{c}}-\left(s\left[{\mathrm{CO}}_{\mathrm{3}}^{\mathrm{2}-}\right]-\mathrm{200}s\right),\end{array}$$

where δ¹⁸O_c is the uncorrected oxygen isotope composition of the carbonate, δ¹⁸O_c^′ is the corrected oxygen isotope composition of the carbonate, s is the selected slope of the effect (in ‰ VPDB per µmol L⁻¹ CO${}_{\mathrm{3}}^{\mathrm{2}-}$), and [CO${}_{\mathrm{3}}^{\mathrm{2}-}$] is the concentration of carbonate ion in solution (in µmol kg⁻¹). This relationship yields no correction when [CO${}_{\mathrm{3}}^{\mathrm{2}-}$] = 200  µmol kg⁻¹, an approximation of the mean modern surface value (after the long-term record of Zeebe and Tyrrell, 2019). The user may specify [CO${}_{\mathrm{3}}^{\mathrm{2}-}$] manually or select a published long-term record of [CO${}_{\mathrm{3}}^{\mathrm{2}-}$] (Tyrrell and Zeebe, 2004; Zeebe and Tyrrell, 2019).

2.5 Tool output

On completion, the tool presents a formatted table of the resulting temperatures, along with any intermediate values (such as estimated δ¹⁸O_w) which were required to generate them. Any rows with potential errors (e.g., paleocoordinates which do not yield a valid δ¹⁸O_w estimate or temperatures which exceed the data range of the calibration) are highlighted in color and flagged with warning text, which appears in an adjacent column. For reference, a short summary of methods is also generated, including relevant equations and a complete bibliography of citations in both text and BibTeX formats for the methods employed in each run.

It should be noted that, while the tool automates the process of applying a given calibration method, the user is still responsible for pre-screening their data for diagenetic alteration or other external biases. For example, use of δ¹⁸O data from foraminifera must consider factors such as diagenetic recrystallization, depth habitat, shell size, and the presence of gametogenic calcite (for a review, see Pearson, 2012).

3 Concluding remarks

Our tool provides a convenient way for workers to perform δ¹⁸O–temperature conversions and explore the sensitivity of their results to different calibrations, corrections, and δ¹⁸O_w reconstruction methods by successively trying different options in the interface. By allowing data generators to rapidly generate multiple temperature estimates for their records with different underlying assumptions, our tool allows workers to quickly understand and quantify the effects of different assumptions on the resulting temperature estimates.