the Creative Commons Attribution 4.0 License.
the Creative Commons Attribution 4.0 License.
CO2-driven and orbitally driven oxygen isotope variability in the Early Eocene
Christopher J. Poulsen
Jiang Zhu
Jessica E. Tierney
Jeremy Keeler
Paleoclimate reconstructions of the Early Eocene provide important data constraints on the climate and hydrologic cycle under extreme warm conditions. Available terrestrial water isotope records have been primarily interpreted to signal an enhanced hydrologic cycle in the Early Eocene associated with large-scale warming induced by high atmospheric CO2. However, orbital-scale variations in these isotope records have been difficult to quantify and largely overlooked, even though orbitally driven changes in solar irradiance can impact temperature and the hydrologic cycle. In this study, we fill this gap using water isotope–climate simulations to investigate the orbital sensitivity of Earth's hydrologic cycle under different CO2 background states. We analyze the relative difference between climatic changes resulting from CO2 and orbital changes and find that the seasonal climate responses to orbital changes are larger than CO2-driven changes in several regions. Using terrestrial δ18O and δ2H records from the Paleocene–Eocene Thermal Maximum (PETM), we compare our modeled isotopic seasonal range to fossil evidence and find approximate agreement between empirical and simulated isotopic compositions. The limitations surrounding the equilibrated snapshot simulations of this transient event and empirical data include timing and time interval discrepancies between model and data, the preservation state of the proxy, analytical uncertainty, the relationship between δ18O or δ2H and environmental context, and vegetation uncertainties within the simulations. In spite of the limitations, this study illustrates the utility of fully coupled, isotope-enabled climate models when comparing climatic changes and interpreting proxy records in times of extreme warmth.
- Article
(15666 KB) - Full-text XML
- BibTeX
- EndNote
The Earth has rapidly warmed since the preindustrial (PI) era, driving substantial and widespread changes in the hydrologic cycle (Douville et al., 2021). Severe warming and changes in the water cycle are projected to continue depending on the level of greenhouse gas emissions. Following a higher emissions pathway, atmospheric CO2 will exceed 1000 ppm by the end of the 21st century, a level that last existed during the Early Eocene about 56–48 million years ago (Tierney et al., 2020). By modeling different orbital and CO2 configurations of the Early Eocene and matching the simulations to fossil evidence, we can provide context for the proxy records and learn how the orbit may have played a part in the severe warming at the onset of the Paleocene–Eocene Thermal Maximum (PETM), as well as different seasonal impacts on the Early Eocene climate. This study serves to distinguish the warming signal from the orbital signal within the hydrologic cycle under the most recent extreme warmth.
The Early Eocene likely experienced atmospheric CO2 concentrations at 3 × PI levels, though proxies range from 500 to 2000 ppm(Anagnostou et al., 2020; Rae et al., 2021). The PETM was a ∼ 100,000 year time interval within the Early Eocene that experienced especially rapid warming, with global surface temperatures rising 5–6 °C in response to an increase in CO2 levels as high as 6 × PI CO2 (Zhu et al., 2019; Inglis et al., 2020; Tierney et al., 2022). Although the geography of the Eocene differs from modern-day geography, simulation of this warming event furthers our understanding of how the Earth operates under a high CO2 background state. There is still much to learn about the Early Eocene and the PETM, especially surrounding the relative influence changes in orbit have on the hydrologic cycle.
Earth's orbital configuration has a strong influence on regional climate and is a driver of major climatic fluctuations (Davis and Brewer, 2011). The orbit determines the timing and intensity of sunlight for a given region and season. Obliquity, the tilt on Earth's axis, has a ∼ 41 000 year cycle; precession, the Earth's wobble, is a ∼ 22 000 year cycle; and eccentricity, the Earth's path around the sun, lasts ∼ 100 000 years. These three factors together determine the solar irradiance any area on Earth will receive at a given time. A higher eccentricity and a higher obliquity cause heightened seasonality, including warmer summer seasons (Davis and Brewer, 2011). Warmer summers often melt more ice, which can accelerate a climatic fluctuation, but the warm Early Eocene lacks a cryosphere, which may have modified the climate's response to warmer summers. Seasonal shifts in solar insolation also drive temperature changes that impact the isotopes of precipitation, and therefore the proxy records related to the isotopic composition of precipitation.
There is evidence of orbital-scale variations in atmospheric CO2 during the Late Paleocene and Early Eocene (Zeebe et al., 2017). Orbitally induced changes in the oceanic temperatures and circulation may have also been a cause for the frequent and variable hyperthermals during the Early Eocene (Lunt et al., 2011; Piedrahita et al., 2022). The PETM was the most extreme hyperthermal, a consequence of even greater atmospheric CO2 concentrations and potentially greater seasonal changes owing to the Earth's orbit.
Oxygen and hydrogen isotopic ratios from meteoric waters are often used as a measure of climate variability, including variability by changing CO2 concentrations or orbit. The ratio of heavy (18O, 2H) to light (16O, 1H) isotopes is represented by 18O or 2H (Craig, 1961). Warmer global temperatures mean more energy in the troposphere to increase evaporation of the heavier isotope – commonly referred to as the temperature effect, and decreased precipitation results in rainfall more enriched in the heavier isotope – commonly referred to as the amount effect, which increases the atmospheric 18O or 2H (Craig, 1961). This can be linked to orbit and atmospheric CO2 because distribution of solar insolation and greenhouse gas concentrations each drive global temperature and precipitation trends, which then influence the 18O or 2H of precipitation (18Op, 2Hp) (Craig, 1961). At a regional scale, oceanic and atmospheric dynamics, like flow of air mass transport and origin of evaporated water, also impact water isotopes.
Although the Early Eocene and PETM have been modeled before, these are the first simulations of that time period to reproduce the extreme warmth and weakened meridional temperature gradient of the PETM and track water isotopes through the hydrologic cycle – which can offer more information on evaporation, advection, precipitation, and other factors that influence 18Op and 2Hp (Zhu et al., 2020). These simulations track water isotopes in every component of the model (Brady et al., 2019). This Early Eocene model has been previously published in Zhu et al. (2019), Zhu et al. (2020), and Tierney et al. (2022). The simulations at varied CO2 have been used to analyze ocean circulation and shortwave cloud feedbacks to further understand parameterizations within the model that play a role in large-scale climate sensitivity (Zhu et al., 2019; Zhu et al., 2020). Additionally, these simulations have been statistically sampled through a data assimilation approach in order to reconstruct PETM climate changes (Tierney et al., 2022). None of these previous studies investigate the exceptional variations in seasonal climate between orbits or the sensitivity of the terrestrial water cycle to both orbital and atmospheric CO2 changes. Through tracking 18Op, this paper underlines the importance of orbital cycles in understanding terrestrial water isotopes.
The biosphere has an impact on water isotopes as well, especially since vegetation patterns likely shifted from the PETM to the Early Eocene. Precipitation infiltrates the soil, is taken up by the roots of plants, and then transpired through leaves. Different plants exhibit different preferential fractionation of water isotopes, which leads to different isotopic signals in the transpired moisture returning to the atmosphere (Gat and Airey, 2006). Plant water oxygen isotope signals can shift by about 6.5 ‰ between the rainy and dry seasons, primarily due to shifts in soil water isotopes (Dai et al., 2020). Quantitative constraints on the plant isotopic effect on atmospheric moisture and precipitation remain difficult to obtain, even more so on a global or regional scale (Gat, 2000). In addition, the vegetation changes for the PETM to Early Eocene are not well constrained, and the estimated fractionation factors for Early Eocene vegetation have high uncertainty (Sachse et al., 2012). Finally, the isotope-enabled land model equipped here assumes the transpired water has the same isotope ratio as the root-weighted soil water (Brady et al., 2019). Therefore, the way water isotopes interact in the biosphere–atmosphere space is primarily based off the soil water, rather than various plant types. The isotope ratio of leaf water is set by the requirement of isotopic mass balance within the plant. There would not be a significant impact on atmospheric water isotope ratios with different vegetation types within the model, as long as the vegetated areas remained vegetated. To that end, these simulations use identical vegetative inputs for each run, isolating CO2 and orbit as controlling factors on changes in water isotopes.
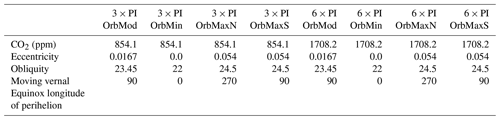
Table 1The atmospheric CO2 concentrations and orbital details are presented here for each modeled case. OrbMod represents a modern orbit, OrbMin represents a minimum eccentricity orbit with mild seasons, and OrbMaxN and OrbMaxS represent maximum eccentricity orbits with heightened seasonality. The longitude of the perihelion is the angle between the Earth at the Northern Hemisphere (NH) autumnal equinox and the Earth at its closest to the Sun: 0° represents a perihelion at the NH autumnal equinox, 90° is at the NH winter solstice, 180° is at the NH vernal equinox, and 270° is at the NH summer solstice. For example, the NH is closest to the sun during the NH summer solstice in OrbMaxN (Fig. A1).
In this study, we use sensitivity experiments to investigate the Early Eocene's climatic and hydrologic response to changes in Earth's orbit and atmospheric CO2 concentration to further understand the impact of orbit on the hydrologic cycle during warm climates and to test Earth's sensitivity to changes in orbit under different CO2 background states. Additionally, we include comparisons between these responses and terrestrial 18O and 2H records to test the model's ability to simulate changes in the hydrologic cycle in an extremely warm climate. The terrestrial data are not constrained to a specific orbit or time of year, so we compare the data to all orbits simulated and the entire seasonal range to determine if the global water isotope signal is captured by the model and to tease out any potential seasonal biases in the data. These analyses strengthen our comprehension of the environmental context of terrestrial proxy records, the potential of orbital changes to partly initiate the hyperthermal, the influence of orbit on the hydrologic cycle at different CO2 forcings, and the potential of this model to simulate climate changes during a time with a higher atmospheric CO2 level than today. We largely find that the orbit may have a more substantial impact on terrestrial water isotope records than atmospheric CO2 in certain regions, particularly in seasonally biased datasets.
2.1 Earth system modeling
The simulations were conducted using a water isotope-enabled Community Earth System Model (iCESM) version 1.2. CESM1.2 is comprised of the Community Atmosphere Model (CAM) version 5.3, Community Land Model (CLM) version 4.0, Community Ice Code (CICE) version 4.0, River Transport Model (RTM), Parallel Ocean Program (POP) version 2, and a coupler to connect them (Hurrell et al., 2013). There are 30 vertical levels in the atmosphere and 60 vertical levels in the ocean. In addition, iCESM has the capability to simulate the transport and transformation of water isotopes in the model hydrologic cycle (Brady et al., 2019). Although there are some noticeable biases in iCESM's isotope tracking, such as a slight depleted bias in 18Op, the model captures the general quantitative features of water isotope movement (Brady et al., 2019). The model resolution is rather coarse at 1.9° × 2.5° for the atmosphere and land, and a nominal 1° for ocean and sea ice. For this reason, we focus on analyzing large-scale, global patterns in the hydrological cycle. The paleogeography, land–sea mask, and vegetation distribution follow the Deep-Time Modeling Intercomparison Project (DeepMIP) protocol at about 55 million years ago (Herold et al., 2014). The ocean temperature and salinity were initialized from a PETM quasi-equilibrated state, and the 18O of seawater was initialized from a constant −1 ‰ to account for the absence of ice sheets in a hothouse climate (Zhu et al., 2020). We completed eight experiments, a control simulation with a modern orbit (OrbMod) and 3 × PI CO2, and seven sensitivity experiments with differing orbital configurations and CO2 levels. There are four orbital configurations, each run at both a low (3 × PI) and high (6 × PI) CO2 concentration, including a modern orbit (OrbMod), maximum summer solar insolation for the Southern Hemisphere (OrbMaxS), maximum summer solar insolation for the Northern Hemisphere (OrbMaxN), and minimum eccentricity (OrbMin) (Lunt et al., 2011). The OrbMaxS and OrbMaxN simulations experience high eccentricity and obliquity, so those simulations would expect high seasonality (Lunt et al., 2011). A control simulation was run for 2000 model years. Orbital cases were branched from the control simulation and run for an additional 500 model years each. The climatological means presented in the results are based on the last 100 years of each simulation. Mean annual climatologies can be found in Figs. A11–A13. All simulations had an atmospheric CH4 concentration of 791.6 ppb and an atmospheric N2O concentration of 275.68 ppb. Atmospheric greenhouse gases other than CO2, like CH4, may have been higher in a warmer climate, but these are kept identical between simulations. Greenhouse gases other than CO2 are poorly constrained for the Early Eocene, and the warming effect on the water cycle is largely captured by the change in atmospheric CO2. CO2 and orbital details for the modeled cases discussed here are available in Table 1. Further details are available in Tierney et al. (2022).
Table 2A list of the proxy records used in model–data comparisons. Paleosol carbonates and siderite are used for a PETM oxygen isotope comparison, and leaf fossils are used for a PETM hydrogen isotope comparison. A terrestrial temperature comparison, reconstructed from macroflora fossil evidence, is used to validate the Early Eocene (3 × PI CO2) simulations in Fig. A2.

2.2 Proxy records
There are more hydrological isotope proxy data from the PETM so that time interval is the focus here rather than the Early Eocene. The 6 × PI CO2 simulations are compared to several terrestrial proxy records of 18O and 2H from the PETM (Table 2). Our focus is on terrestrial proxies over marine proxies as terrestrial proxies are more heavily impacted by changing orbit and seasonality, given the land-ocean warming contrast (Byrne and O'Gorman, 2013). However, the terrestrial data are not dated to a specific orbit or season given uncertainties in the dating relative to orbital pacing, so we use it as an approximate envelope of PETM water isotope values against the range of values from all simulated orbits and seasons. Zhu et al. (2019) previously showed good model–data agreement between terrestrial temperature proxies and the Early Eocene (3 × PI CO2) simulation, validating the model's performance (Fig. A2, Zhu et al., 2019). These Eocene simulations also show strong agreement with marine proxies, as discussed in Zhu et al. (2020). The suite of Eocene simulations run as part of the Paleoclimate Modeling Intercomparison Project exhibit close agreement with terrestrial precipitation proxies as well (Cramwinckel et al., 2023).
Paleosol carbonates and siderite preserve the isotopic signal of the soil water they form in, thus a comparison with simulated soil water, rather than precipitation, is the most salient comparison. Soil carbonates are likely to form around 40–100 cm deep, so the simulated 18O range represents soil water at this depth as well (Burgener et al., 2016). In order to compare the proxy 18O to simulated soil water 18O, a proxy system model (PSM) is needed to transform 18O of the soil carbonate to 18O of the water in which they precipitated from. The fractionation factor is controlled by the local soil temperature, so each location has a slightly different fractionation factor, though all are near 1 (van Dijk et al., 2018; Friedman and O'Neil, 1977). Siderite oxygen fractionation was calculated through Van Dijk's best fit equation (van Dijk et al., 2018). The paleosol carbonate's oxygen fractionation was calculated through the USGS equation (Friedman and O'Neil, 1977).
Leaf waxes are another powerful tool for paleoclimate reconstruction and also require a PSM to account for the transformations between the 2H of soil water and the δ2H within the leaf wax (Konecky et al., 2019a). Therefore, the δ2H model–data comparison includes δ2H from PETM leaf waxes and model-inferred leaf wax δ2H seasonal ranges. Comparing leaf wax δ2H in addition to the soil 18O proxies offers an opportunity to explore hydrogen isotope accuracy within iCESM. The model-inferred leaf wax 2H values were calculated through the WaxPSM using the zonal seasonal range of soil water 2H and a global, fixed apparent fractionation factor of −124 ‰ (Konecky et al., 2019b). The apparent fractionation factor differs between different plants, but it is unknown for Early Eocene vegetation, so this study uses an average value for a modern landscape that is equal parts shrubs, trees, forbs, and C3 grasses (Sachse et al., 2012).
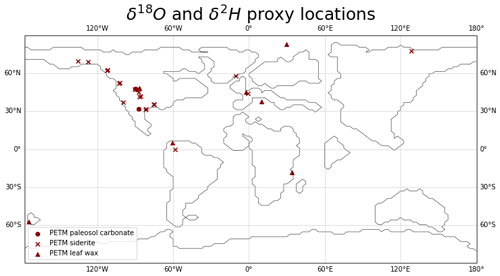
Figure 1The Early Eocene paleogeography used for the model simulations, with points representing the locations of the PETM terrestrial isotope proxy record. Note that two of the records originated on islands that do not appear on this paleogeographic map. There is a noticeable lack of paleo-isotope records from the SH.
The soil carbonate proxies act as a time-integrated record of environmental changes over hundreds to thousands of years, while the leaves form over a matter of weeks (Burgener et al., 2016). Although the proxy records span a wide geographic range, we could not find published terrestrial 18O proxy records for the PETM from the Southern Hemisphere (SH), which signals a need for more focus and funding to be directed to researchers in the SH to fill this gap. The locations of the proxies were converted to paleo-coordinates suitable for our paleogeographic reconstruction (Fig. 1, Muller et al., 2018).
3.1 Response to orbit
High eccentricity and obliquity cause more intense seasonality (Berger, 1988). We focus our analysis on the OrbMaxS and OrbMaxN simulations because their large seasonal insolation variations drive the greatest terrestrial climate response (Fig. A1). OrbMaxS and OrbMaxN are at the same (high) eccentricity and obliquity as one another, so these differences are the result of precessional changes only (Table 1). Section 3.1 focuses on response to orbit at the 3 × PI CO2 level because there were greater climatic differences between orbits at this level (see Sect. 3.3).
Differential solar heating, controlled by orbital forcing, gives rise to a latitudinal temperature gradient and has a great influence on Earth's climate. Insolation changes drive differences in specific humidity between these runs, since warmer air can hold more water vapor with an increase in saturation vapor pressure (SVP) (Fig. A4). However, areas that experience an increase in temperature and specific humidity, like central Africa or Australia during DJF, largely experience a decrease in relative humidity, the ratio of water vapor to the SVP, as SVP increases more than specific humidity during warming (Fig. A5, Tichy et al., 2017). Lower relative humidity results in a lower chance of cloud formation and rainfall, which often only occurs when relative humidity is > 90 %. With a lower chance of precipitation, these areas are less likely to experience isotopic rainout of the heavy isotope and therefore often exhibit higher 18Op (Figs. 3 and 4).
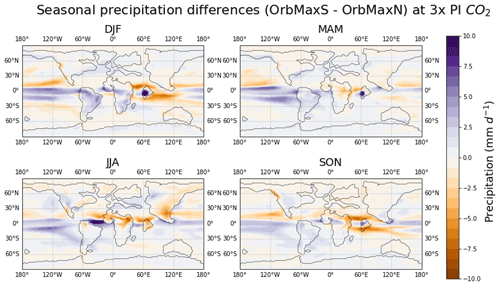
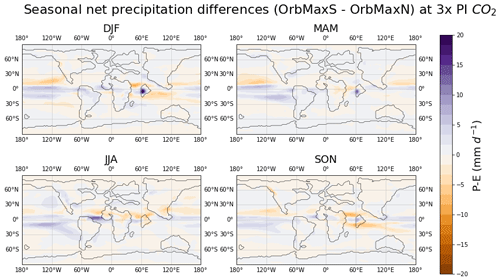
Figure 3The seasonal precipitation differences between OrbMaxS and OrbMaxN at 3 × PI CO2. The maximum positive precipitation difference on the corresponding color bar represents anything experiencing 10 mm d−1 or higher.
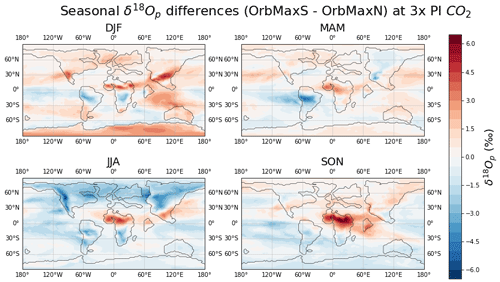
Both 18Op and 2Hp exhibit large-scale seasonal differences between OrbMaxS and OrbMaxN; DJF (JJA) experiences greater (lesser) 18Op and 2Hp in OrbMaxS than OrbMaxN driven by the difference in seasonal insolation (Figs. 4 and A6). The increase in insolation during DJF also encourages stronger evaporation rates from the sea surface, which is influential on isotopic signals as the origin of transported moisture and sometimes encourages continental recycling through evapotranspiration (Figs. 4 and A16; Gierz et al., 2017; Risi et al., 2019). With higher insolation and lower relative humidity, the air is generally drier and able to stimulate higher rates of evaporation (Fig. A5). Therefore, the DJF season experiences higher insolation, warmer temperatures, higher rates of evaporation, and generally a stronger presence of heavier isotopes in atmospheric moisture and, in turn, precipitation (Figs. 2–4, A3, and A5). JJA experiences a decrease in insolation, lower temperatures, lower rates of evaporation, and generally a weaker presence of heavy isotopes in precipitation (Figs. 2, 4, A1, and A5). Globally, the large-scale simulated 2Hp patterns mimic the large-scale simulated 18Op patterns, since the fractionation of hydrogen and oxygen are controlled by the same distillation factors (Fig. A6).
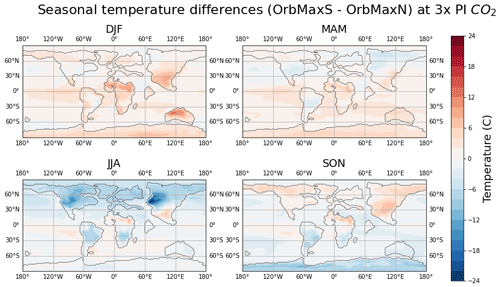
Aside from the large-scale isotopic signals, specific regions experience large seasonal differences in 18Op with a change in orbit. For instance, western North America sees a substantial 18O depletion in precipitation during JJA when comparing OrbMaxS to OrbMaxN, up to −5 ‰ (Fig. 4). JJA is the SH winter season, so most of the Earth is colder at this time in the OrbMaxS simulations, especially regions of high elevation like the North American Cordillera, which likely had an elevation upwards of 3 km in some areas (Fig. 2, Mulch et al., 2007). The decrease in temperature resulted in an increase in relative humidity in the area, increasing rainfall (Figs. 3 and A5). Mountains already intensify vapor fractionation, reducing the 18Op through the amount and temperature effects, so the Cordillera experienced especially depleted rainfall during JJA for the OrbMaxS simulations compared to the OrbMaxN simulations, which experienced a very hot NH during JJA (Fig. 4, Poulsen et al., 2007). Evaporated water from the cool Pacific Ocean travels in the prevailing westerly winds over continental North America. As the moisture ascends the mountainside, there is increased rainfall, further depleting the clouds of the heavier water isotopes, and leading to a dry and isotopically light descending air mass (Fig. 4). Furthermore, regions like northern Africa or the Tibetan Plateau experience increases in temperature and decreases in precipitation year-round, as well as increases in 18Op mostly due to temperature and amount effects (Figs. 2–4). Northern Africa experiences enriched rainfall during all seasons, especially SON, with differences up to ∼ 6.5 ‰ between OrbMaxS and OrbMaxN (Fig. 4). Temperatures are warmer, and relative humidity and rainfall rates are lower, resulting in substantially enriched rainfall (Figs. 2–4, and A5). High rates of evaporation from the warm pool accompany the trade winds to transport relatively isotopically heavy moisture to the primarily warm and dry Sahara Desert region (Figs. A5 and A16). This region has sparse vegetation and resulting low rates of evapotranspiration, so the water isotopes in precipitation are largely consequence of the evaporative source – the nearby seawater. The cooler, drier wind above the Indo-Pacific during SON passes over the warm sea and evokes higher evaporation rates due to the strong gradients in temperature and moisture between the air–sea surfaces, and that enriched air mass is quickly swept away towards the nearby land mass. The little rainfall this region experiences is therefore isotopically heavier in the OrbMaxS simulation (Fig. 4).
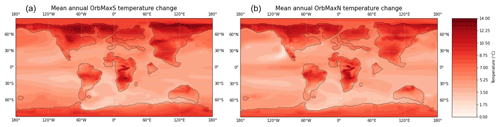
Figure 5The difference in mean annual surface temperatures at 6 × PI CO2 compared to 3 × PI CO2 for OrbMaxS (a) and OrbMaxN (b). To view absolute mean annual surface air temperatures, see Fig. A11.
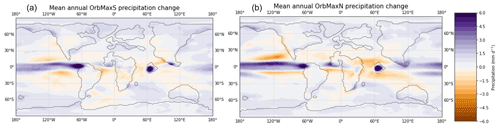
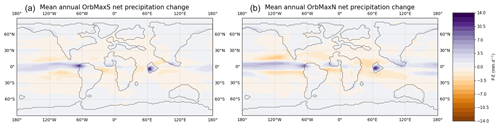
Figure 6The difference in mean annual precipitation under 6 × PI CO2 compared to 3 × PI CO2 for OrbMaxS (a) and OrbMaxN (b). The maximum positive precipitation difference on the corresponding color bar represents anything experiencing 6.0 mm d−1 or higher. To view absolute mean annual precipitation, see Fig. A12.
3.2 Response to CO2
Past studies have shown that simulated climate responds strongly to changes in atmospheric CO2 levels (Poulsen et al., 2007). Here, we compare the climatic CO2 response for different orbits. Increasing greenhouse gases results in warmer global temperatures, an enhanced hydrologic cycle, and 18O enrichment over land (Figs. 5 and 7). Section 3.2 shows hydrological and 18Op responses to increased CO2 for both OrbMaxS and OrbMaxN. Seasonal partitioning did not reveal any further insights, so we focus on annual-average results.
As a consequence of the increase in CO2, globally averaged surface temperatures increase by ∼ 6 °C in the 6 × PI CO2 simulations compared to the 3 × PI CO2 simulations (Fig. 5). There is a greater rise in temperature over land than ocean – an average 41 %–44 % increase in surface temperature over land compared to an average 17 %–19 % increase in surface temperature over ocean, largely owing to the land–sea contrast in heat capacities (Fig. 5, Dong et al., 2009). Land near the Arctic generally warms the most and sees a slight increase in precipitation (Figs. 5 and 6). The increased temperatures produce higher rates of evaporation for the heavier oxygen isotope and increases the residence time of water vapor in the atmosphere, which may contribute to an increased advective length scale of enriched moisture transport (Singh et al., 2016). Decreased relative humidity leads to decreased rates of precipitation – specifically in the subtropics at the dry, descending region of the Hadley Cell, which result in increased 18Op in the 6 × PI CO2 simulations (Figs. 6, 7, A8, and A17). There is also an increase in sea surface temperatures and evaporation over these subtropical warmer waters which populate the air mass with heavier oxygen isotopes and increase 18Op (Figs. 7 and A17).
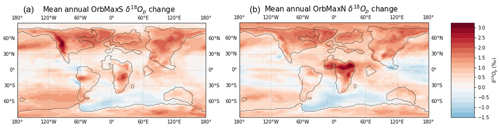
Figure 7The difference in mean annual 18Op at 6 × PI CO2 compared to 3 × PI CO2 for OrbMaxS (a) and OrbMaxN (b). To view absolute mean annual 18Op, see Fig. A13.
Though most areas exhibit an increase in 18Op with the 6 × PI CO2 modeled case, the western equatorial Pacific and some subpolar regions show a slight decrease (Fig. 7). These regions experience increases in relative humidity, cloud cover, and precipitation under the higher CO2 background state, resulting in 18O-depleted rainfall (Figs. 6, A7, and A8). The colder subpolar region does not experience much of an increase in surface air temperatures, ∼ 2 °C or less, which may have contributed to the slight increase, ∼ 3 % or less, in relative humidity (Figs. 5 and A8). The equatorial Pacific sees an increase in temperature, but it also experiences highly increased rainfall (Fig. 6). There has been previous evidence of a narrowing and strengthening of the Inter-Tropical Convergence Zone (ITCZ) during the onset of the PETM, which would increase precipitation, resulting in decreased 18Op (Tierney et al., 2022; Cramwinckel et al., 2023; Byrne and Schneider, 2016). The narrowing tendency is largely due to the enhanced meridional moist static energy gradient seen in warming climates with increased atmospheric moisture (Fig. 6; Byrne and Schneider, 2016). Moreover, there is often a widening of the Hadley circulation projected in warming climates, which contributes to the expansion of a dry descent region off the tropics (Figs. 6 and A17; Byrne and Schneider, 2016). With stronger, unsaturated downdrafts, there is an expected increase in 18Op over most subtropical land (Fig. 7).
The North American Cordillera experiences large increases in 18Op under the higher CO2 conditions (Fig. 7). Areas of high elevation generally have very low 18Op compared to areas of low elevation due to isotopic distillation during rainout. This elevation distinction is reduced during times of extremely warm temperatures due to atmospheric subsidence of vapor enriched in 18O, which explains the substantial increase, up to 3 ‰, in 18Op with a doubled CO2 (Fig. 7, Poulsen and Jeffery, 2011). However, OrbMaxN does not see as dramatic an increase in 18Op under higher CO2 conditions at this mountain range (Fig. 7). The isotopic response is lower because the higher insolation in the NH at OrbMaxN already caused a response under the 3 × PI CO2 conditions (Fig. A13). The 18Op over the mountain range is higher in the OrbMaxN case than the other cases at 3 × PI CO2 because the increased insolation warmed the mountain range and increased 18Op substantially, so there is a smaller difference in 18Op between the CO2 levels (Fig. 7).
Finally, most orbits display just a slight increase in 18Op in northern Africa under the higher CO2 state, but OrbMaxN experiences a substantial increase in 18Op in northern Africa, up to 3 ‰, largely owing to drier conditions, including over the Indo–Pacific warm pool where most of the moisture is sourced (Figs. 6, 7, A8, and A17). Northern Africa experiences a stronger decrease in relative humidity for OrbMaxN than any other orbit under the higher CO2 state (Fig. A8). The decrease in relative humidity and precipitation results in more enriched rainfall causing a stronger increase in 18Op over this desert and shrubland region under OrbMaxN conditions (Fig. 7). Additionally, the prevailing trade winds are also relatively cooler and drier over the Indo–Pacific in OrbMaxN, though the Indo–Pacific warm pool remains very warm under all orbits, resulting in a stronger temperature and moisture gradient at the air–sea interface. This gradient increases evaporation rates at the source, resulting in higher 18Op over most of the Sahara (Figs. 7, A8, and A17).
3.3 Orbital sensitivity under different CO2 background states
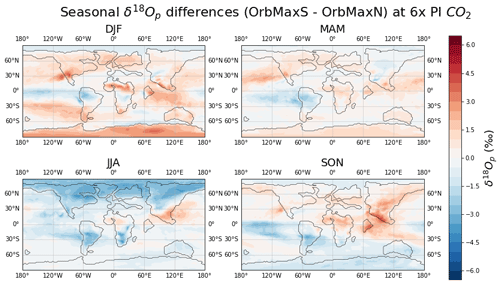
Given the above results, the hydrologic cycle is clearly impacted by both changes in orbit and changes in atmospheric CO2 levels. However, none of the above results address the potential difference in the hydrologic cycle's orbital sensitivity under two different CO2 background states. In other words, do changes in orbit at a lower CO2 level have a greater impact on the oxygen isotopic ratio of precipitation than changes in orbit at a higher CO2 level? When comparing the 18Op differences between OrbMaxS and OrbMaxN at 3 × PI CO2 versus 6 × PI CO2, the spatial patterns of enrichment and depletion of heavy oxygen isotopes are similar (Figs. 4 and 8). However, the global mean annual 18Op difference between these two orbits is over 20 % smaller for 6 × PI CO2 than 3 × PI CO2. Averaged globally, the change in orbit has a smaller impact on the hydrologic cycle at the higher CO2 level, representative of the PETM.
Additionally, the regions that experience a larger enrichment or depletion of heavy oxygen isotopes between orbits during certain seasons, like western North America or northern Africa, see a much smaller 18Op difference at the higher CO2 level than the lower CO2 level, by as much as 4 ‰ (Figs. 4 and 8). Therefore, the higher atmospheric CO2 level dampens the orbital sensitivity of the hydrologic cycle, especially in these regions. Fractionation of oxygen isotopes is more pronounced at lower temperatures, and the 3 × PI CO2 background state exhibits much lower global temperatures than the 6 × PI CO2 background state (Fig. 5, Luz et al., 2009). CO2-induced warming tends to slow general circulation in the tropics and subtropics (Singh et al., 2016). Higher temperatures result in higher rates of evaporation, an increase in water vapor residence time, and more 18O in the atmosphere, which causes a lower fractionation factor between the lighter and heavier oxygen isotopes and less rainout and distillation (Luz et al., 2009). The smaller fractionation rate between oxygen isotopes during evaporation and decrease in rainout and distillation results in smaller, muted differences in the oxygen isotope variability of precipitation between orbits. Therefore, the change in insolation distribution has less of a considerable impact on the hydrologic cycle at higher atmospheric CO2 levels.
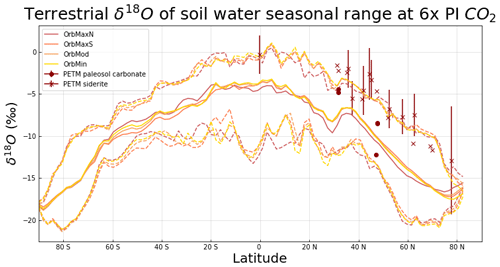
Figure 9The simulated seasonal range of soil water 18O at a depth of 40–100 cm for each orbit under 6 × PI CO2 conditions compared to PETM paleosol carbonate and siderite 18O records. The middle solid lines represent the mean annual soil water 18O at each latitude for all terrestrial longitudes, and the lower and upper dashed lines represent the lowest and highest monthly means at each latitude, respectively. The error bars represent uncertainty. The specific summer and winter means can be found in Fig. A9.
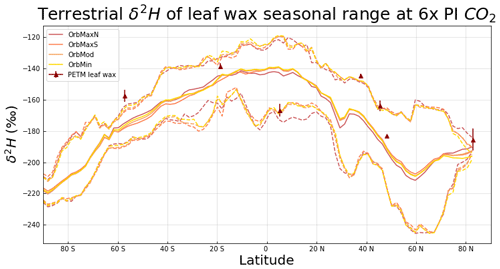
Figure 10The seasonal range of model-inferred leaf wax 2H for each orbit under 6 × PI CO2 conditions compared to PETM leaf wax 2H records. The middle solid lines represent the mean annual leaf wax 2H at each latitude for all terrestrial longitudes, and the lower and upper dashed lines represent the lowest and highest monthly means at each latitude, respectively. The error bars represent uncertainty. The specific summer and winter means can be found in Fig. A10.
3.4 Model–data comparison
Isotope-enabled climate models can simulate the transformation and transportation of water isotopes in all components of the model, which allows for direct comparison between modeled isotopic ratios and paleo-recorded isotopic ratios and further assessment of uncertainties (Zhu et al., 2017). This study gathers 18O data from soil carbonates and δ2H data from leaf waxes in order to validate iCESM's simulated terrestrial hydrologic cycle during the PETM, which had more available data than the Early Eocene. The lower CO2 background state, representative of the greater Early Eocene age, is validated through a terrestrial temperature model–data comparison (Fig. A2, Zhu et al., 2019). The isotopic model–data comparison results focus only on the 6 × PI CO2 simulations and PETM records. The comparisons account for seasonality by including both the highest and lowest mean monthly soil water 18O or 2H (dashed lines) for all terrestrial longitudes at the given latitude for each orbital simulation, along with the mean annual values (solid lines) (Figs. 9 and 10). These figures concisely capture the entire seasonal range of simulated isotopic signals latitudinally. To account for regional effects rather than global effects, we also produce a point-by-point comparison of proxy isotopic data with simulated isotopic data at the grid cells in the model that correspond with the paleo-coordinates of each proxy record (Figs. A14 and A15). These figures include mean annual and mean summer isotopic data to investigate potential seasonal biases in the data, as well as biases within the model.
About 60 % of PETM soil carbonate records fall within the simulated seasonal range for soil water 18O, and several siderite records fall above the highest monthly means, which likely represent the warm season (Fig. 9). In order to capture as many proxy records as possible and not assume a particular seasonal time of formation, Fig. 9 displays the largest possible range by utilizing the lowest and highest monthly modeled soil water 18O at each latitude. However, we also created the same figure using specifically summer and winter means, though the range becomes tighter (Fig. A9). The siderite record may be warm season biased, and the model may exhibit a slightly low 18O bias. Further assessment of model and proxy biases contributing to this occurrence can be found in Sect. 4.
About 70 % of PETM leaf wax records fall within the simulated seasonal range for model-inferred leaf wax 2H (Fig. 10). In order to capture as many proxy records as possible and not assume a particular seasonal time of formation, Fig. 10 displays the largest possible range by utilizing the lowest and highest monthly model-inferred leaf wax 2H at each latitude. However, we also created the same figure using specifically summer and winter means, though the range becomes tighter (Fig. A10). Records outside of the largest range may be seasonally biased or have a different fractionation factor than the model-inferred values. Further assessment of model and proxy biases contributing to this occurrence can be found in Sect. 4.
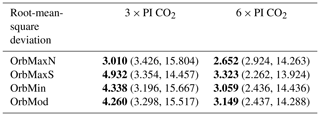
The PETM was a relatively short period of extreme warming, and the eccentricity configuration would have remained almost constant during the onset. Furthermore, orbit has a substantial influence on climate, so a best guess as to the orbit during the onset of the PETM would impact our understanding of the PETM global warming and how it compares to present-day global warming. Figures 9 and 10 fail to emphasize orbital differences, as they only display zonal seasonal range, so this study employs a quantitative point-by-point comparison method to emphasize orbital differences. The simulated 6 × PI CO2 18Op values are compared to the Van Dijk et al. (2020) siderite PETM record to evaluate which orbital simulation is the best match. This record includes the widest span of latitudes and longitudes and analyzed several samples at each location to find a mean 18O value. In order to practically compare these proxy values to the simulated values, we found which group of four model grid cells captured the paleo-coordinates of each proxy location, and then found the NH summer mean 18O values for that group of cells for each simulation. The siderite proxy values originate from the NH and appear to represent summer values (Table A1, Figs. 9 and A14). The modeled summer days have been adjusted for the paleo-calendar effect, which structures time as a fixed number of degrees in Earth's orbit, rather than a fixed number of days each month, so that seasonal comparisons between simulation and data are properly lined up according to the Earth's position in its orbit (Bartlein and Shafer, 2019). The simulated 18O summer means at each of the 10 locations were then used to calculate the root-mean-square deviation (RMSD), calculated following Eq. (1):
RMSD is commonly used for model–data comparisons (Flato and Marotzke, 2013; Thompson et al., 2022). The lower the result, the more comparable the simulated values are to the proxy values. The 6 × PI CO2 and OrbMaxN run is in best alignment with the proxy record. However, we are limited by the lack of SH Van Dijk records representing the PETM. The Van Dijk RMSD values are in bold (Table 3). We also decided to complete the RMSD with the other 18O records (first number in parentheses), as well as the 2H records (second number in parentheses), to see if their pattern of comparability would be consistent with the Van Dijk siderite record. All proxy records are more comparable to the 6 × PI CO2 simulations than the 3 × PI CO2 simulations since they are all from the PETM, but the orbital preferences varied (Table 3). Other hesitations, drawbacks, and speculations can be found in Sect. 4.
These Early Eocene sensitivity experiments provide a window to large-scale climate patterns and paint some environmental context for proxy records. Our model simulated four orbits at two different atmospheric CO2 settings and traced water isotopic ratios to allow for comparisons to various water isotope proxy records. A past study using a lower resolution model of the Early Eocene found a heavy influence of orbit on seasonal precipitation trends during the Early Eocene and our findings agree (Keery et al., 2018). OrbMaxS and OrbMaxN simulations exhibit high eccentricity and obliquity, resulting in intense seasonality, but different orbital precession, resulting in dramatic differences in insolation distribution seasonally (Fig. A3).
We find dramatic differences in 18Op between OrbMaxS and OrbMaxN as a result of seasonal changes in insolation, reaching up to ∼ 6.5 ‰ in certain regions (Fig. 4). The differences in 18Op between the two CO2 background states were less dramatic, reaching only ∼ 3.0 ‰ in certain regions (Fig. 7). Key regions of interest (those experiencing the greatest differences in oxygen isotopic signals) show greater changes in 18Op in response to orbital change than a CO2 doubling due to higher seasonal sensitivity. This may be key to explaining some variability within terrestrial water isotope paleo-records. For instance, the variation in the terrestrial 2H leaf record in Inglis et al. (2022) may be partly attributed to orbital variability. However, the simulations with doubled CO2 showed a consistent, mean annual increase in 18Op, which orbital changes are less likely to provoke. Therefore, changes in precession play an important role in the hydrologic cycle seasonally and present a valuable piece of information to consider when interpreting paleo-records – especially when those records form over shorter periods of time and are seasonally biased.
Additionally, we find that orbital variability has relatively greater influence on precipitation isotopes under a lower CO2 condition. This may imply that orbit exerts more control on the seasonal hydrological cycle in colder climates than warmer climates. As such, it may be especially important to incorporate the potential influence of orbital variability on colder, long-interval climate studies in future work. Studying orbital control on the hydrological cycle in warmer climates is still recommended, but it may have a slightly less considerable impact in extremely high CO2 environments.
Some paleosol carbonate proxies most closely match maximum simulated soil water 18O, which represents summer values, though not all (Fig. 9). This comparison is consistent with the idea that paleosol carbonates sometimes preserve a signal of the isotopic composition of rainfall during the warmer, more evaporative season in which the carbonates may be more likely to form (Kelson et al., 2020). The siderite records appear to fall along the maximum 18O simulated values for all modeled cases, signaling a possible warm-season bias. Recent studies argue that pedogenic siderite forms between the mean annual air temperature and the mean air temperature of the warmest months, depending on the latitude (Fernandez et al., 2014; van Dijk et al., 2019). Some temperature reconstructions support that siderite forms more rapidly under warmer, more evaporitic conditions, especially in higher latitudes, since it is controlled by microbial iron reduction which proceeds faster in higher soil temperatures (van Dijk et al., 2020). Therefore, both archives likely have some records that represent the soil water 18O during the warm, dry, evaporative season when they are more likely to form, but especially the siderite. As siderite appeared consistently warm season biased, we chose to use the summertime average soil temperature for the siderite PSM. The summer season receives the greatest insolation, which increases temperature and evaporation rates, which in turn would have biased the isotope recording if this environment did encourage faster soil carbonate growth. This bias is seen in the point-by-point comparison as well, which highlights regional climate over global climate, since the simulated mean summer isotopic signals more closely mimic the proxy data than the simulated mean annual isotopic signals (Figs. A14 and A15).
The terrestrial 18O proxy records span much of the NH, but are lacking in the SH, limiting our model–data comparison. Although the model's paleo-elevation roughly matches the paleo-elevation estimates from the proxy records, proxies from the highest elevations were excluded because paleo-altimetry estimates have larger uncertainty. Aside from the seasonal bias, the exclusion of high-elevation proxies may explain why none of the records sit closer to the minimum 18O values, as areas of high elevation often result in low 18O, though this is not necessarily the case under high CO2 conditions (Dansgaard, 1964). Additionally, there is evidence that iCESM1.2 has a slight low bias for 18Op, which may transfer to the 18O of soil water and drive further misalignment between model and proxies (Brady et al., 2019). This slight low bias may partially explain the misalignment between simulated and proxy isotopic signals that is seen in the point-by-point comparison as well (Figs. A14 and 15). The model also exhibits some depleted bias in 2H, as well as the presence of a double ITCZ, which may encourage biases in extratropical moisture transport (Brady et al., 2019). Other limitations in this model–data comparison may include the possibility of evaporation before burial, diagenesis, uncertainty in timing, varying elevations, or error in paleo-coordinate conversion or fractionation factor.
Furthermore, the terrestrial 2H proxy records largely fall within the simulated seasonal range for model-inferred leaf wax 2H (Fig. 10). The Jaramillo et al. (2010) record is closer to the minimum 2H, likely because that record was taken from a tropical rainforest with increased and 2H-depleted rainfall, but the other records align closer to mean or maximum 2H (Fig. 10, Jaramillo et al., 2010). Leaves undergo transpiration while growing, an evaporative process that further fractionates water isotopes, which often result in 2H-enrichment of leaf water and has a critical effect on the final 2H of leaf wax n-alkanes (Kahmen et al., 2013). Therefore, it is likely that some records align closer to maximum 2H values in part due to transpiration. Leaves also tend to grow over a matter of weeks, so seasonal bias and short-term formation could be another reason for slight mismatches between the model and data. Plus, the fractionation factor used in the WaxPSM is a globally averaged estimate, and there is a wide range of potentially realistic fractionation factors that could shift leaf wax 2H values by as much as ∼ 20 ‰ (Handley et al., 2011; Pagani et al., 2006). The Earth experienced a major precession-driven modification of global vegetation during the PETM and across the Eocene, so the changes in biosphere–atmosphere interactions and plant biology could have significantly impacted the hydrological cycle and leaf wax isotopes (Tardif et al., 2021). Aside from that, some of the same limitations faced in the 18O comparison are also relevant in the 2H comparison – diagenesis, uncertainty in timing, varying elevations, or error in paleo-coordinate conversion. However, the majority of leaf wax records fall within the model-inferred seasonal range of leaf wax 2H.
Finally, previous studies indicated that Earth experienced near-maximum solar irradiance at the onset of the PETM largely due to variations in eccentricity (Zeebe et al., 2017; Lourens et al., 2005; Westerhold et al., 2009; Zachos et al., 2010; Galeotti et al., 2010; Westerhold et al., 2012). Several studies argue that the Earth's eccentricity at this time may have partly caused the extreme warming during the PETM, and our findings agree (Kiehl et al., 2018; Lawrence et al., 2003). The Kiehl et al. (2018) study also argues that the Earth was likely experiencing an orbit most similar to OrbMaxN at the onset of the PETM (Table 3). Although it is worth constraining the orbit at the onset of the PETM in order to further understand the relatively rapid and extreme warming that followed, there are several limitations to this model–data comparison that render it less effective. There are biases with the oxygen isotope records, discussed above, and several drawbacks of the simulations, including the resolution and model bias. These model simulations are run with a relatively coarse atmosphere (∼ 2° horizontal resolution) and topography, which may not fully capture the local environments of the proxy records. Perhaps most importantly, the timing of the onset of the PETM is not perfectly constrained so the proxy records may not represent the onset itself. So aside from the van Dijk siderite record, we also conducted an RMSD for the other PETM proxy records to speculate on what consistent (or inconsistent) patterns between simulations could mean. All records match the higher CO2 level better than the lower CO2 level within the same orbit, which was expected with PETM records (Table 3). However, the other 18O records did not match OrbMaxN best but instead matched OrbMaxS best at the PETM greenhouse gas level. The 2H records seem to favor OrbMaxS as well if anything. Given the variability within the RMSD, we hesitate to draw any strong conclusions, but we speculate that the records all likely formed during varying orbits or were slightly more likely to form during orbital times of maximum seasonality.
Determining the potential orbit in existence at this time can contribute to our knowledge of how this past global warming differs from our present-day global warming. For instance, the 6 × PI CO2 and OrbMaxN modeled run is relatively far from our present-day warming scenario. The current atmospheric CO2 concentration is about one-quarter as high, and the 6 × PI CO2 OrbMaxN simulation has a mean annual global temperature 0.71 °C higher than the 6 × PI CO2 OrbMod simulation, largely driven by the higher insolation maximum of OrbMaxN. If the 6 × PI CO2 OrbMaxN simulation is the closest to representing the onset of the PETM, this highlights how the PETM differs from modern climate in important ways that constrain its use as an analogue for the Anthropocene, especially since a maximum NH summer insolation orbit would have slightly bolstered global warming during the PETM, unlike our modern orbit.
This study demonstrates the relative impact of CO2 and orbit on climate and the orbital sensitivity of the hydrologic cycle under different CO2 background states through the combination of a fully coupled climate model suite of the Early Eocene and published terrestrial 18O and 2H proxy records. Our results reveal that large variations in orbit can have a more substantial impact on the hydrologic cycling than doubling CO2 in certain regions seasonally, although doubling CO2 has a more consistent and global-scale impact, and that orbital sensitivity weakens under a higher CO2 background state. These findings highlight the importance of modeling various orbital states to understand variation in water isotope records and stress the influence changes in orbit have on seasonal climate relative to changes in greenhouse gases. This study also determines that the concentration of greenhouse gases in the atmosphere partly controls the sensitivity of the climate to orbital changes. The comparison to terrestrial 18O and 2H records verifies the reliability of our model and lends some interpretation to the biases within iCESM and the environmental context pertaining to the potential warm-season bias of some of the proxies so we may better understand what they represent. The iCESM generally performs well in simulating hydrologic cycling during a warmer climate, which increases trust in iCESM to project future climate change, though some water isotope biases exist within the realm of the model. The empirical evidence appears to host biases as well, especially siderite with a seasonal bias, which underscores the importance of taking orbit into account when assessing siderite records. Exploring OrbMaxN as a potential cause for the onset of the PETM is worthwhile, though many uncertainties in the model–data comparison exist. Simulating the Early Eocene is valuable as it experienced high CO2 levels, heightened seasonality, and precipitation extremes, which we expect with future climate change. These simulations further our understanding of the role CO2 and orbit play in climate change and the hydrologic cycle.
Table A1The modern and paleo-coordinates for each van Dijk et al. (2020) proxy location, followed by the appropriate model grid cells and site names.
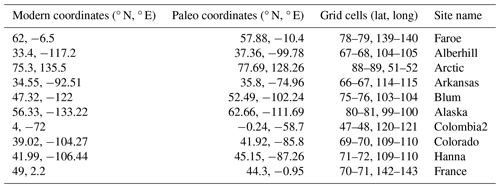
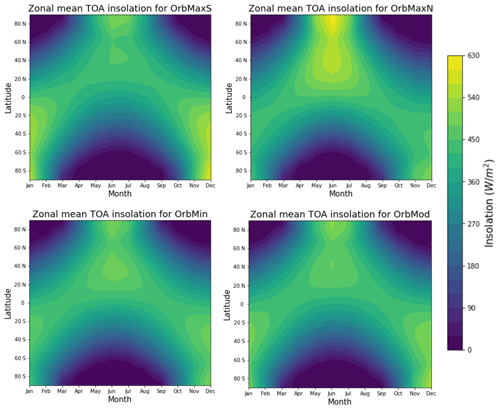
Figure A1The zonal, monthly top-of-atmosphere (TOA) solar insolation distribution for all orbits. OrbMaxS and OrbMaxN reach a higher summer insolation than OrbMod or OrbMin.
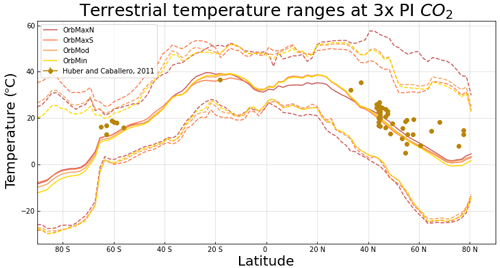
Figure A2Model–data comparison between the Huber and Caballero (2011) Eocene terrestrial dataset and the 3 × PI CO2 simulations. The middle solid lines represent the annual mean at each latitude for all terrestrial longitudes, and the lower and upper dashed lines represent the lowest and highest monthly means at each latitude, respectively.
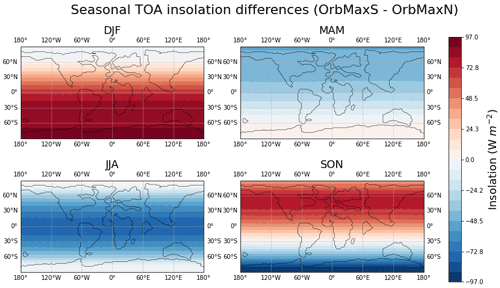
Figure A3The seasonal zonal top-of-atmosphere (TOA) solar insolation difference between OrbMaxS and OrbMaxN.
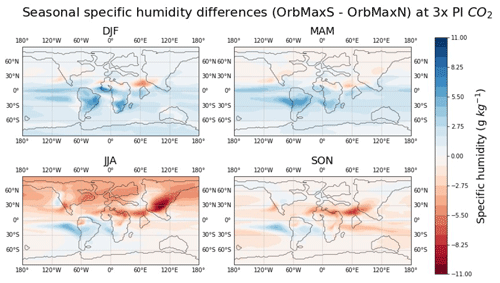
Figure A4The seasonal specific humidity differences between OrbMaxS and OrbMaxN at 3 × PI CO2. Specific humidity is presented here at 850 atmosphere hybrid sigma pressure coordinates. Sigma coordinates represent pressure at the Earth's surface rather than the mean sea level, so it follows the actual terrain. We chose these coordinates because they represent the same pressure level above the land without running into mountains.
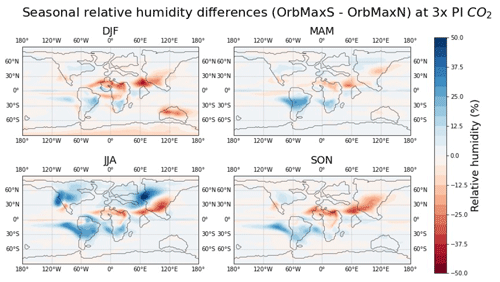
Figure A5The seasonal relative humidity differences between OrbMaxS and OrbMaxN at 3 × PI CO2. Relative humidity is presented here at 850 atmosphere hybrid sigma pressure coordinates. Sigma coordinates represent pressure at the Earth's surface rather than the mean sea level, meaning it follows the actual terrain. We chose these coordinates because they represent the same pressure level above the land without running into mountains.
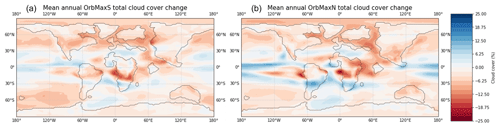
Figure A7The difference in mean annual total cloud coverage under 6 × PI CO2 compared to 3 × PI CO2 for OrbMaxS (a) and OrbMaxN (b).
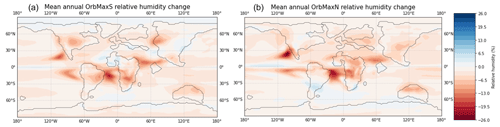
Figure A8The difference in mean annual relative humidity at 6 × PI CO2 compared to 3 × PI CO2 for OrbMaxS (a) and OrbMaxN (b). Relative humidity is presented here at 850 atmosphere hybrid sigma pressure coordinates. Sigma coordinates represent pressure at the Earth's surface rather than the mean sea level, so it follows the actual terrain. We chose these coordinates because they represent the same pressure level above the land without running into mountains.
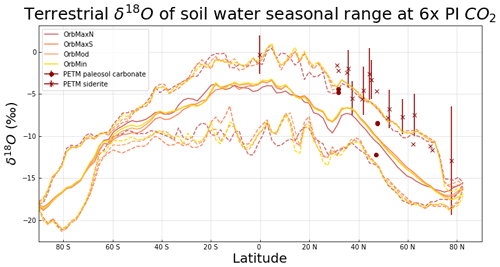
Figure A9The simulated seasonal range of soil water 18O at a depth of 40–100 cm for each orbit under 6 × PI CO2 conditions compared to PETM paleosol carbonate and siderite 18O records. The middle solid lines represent the mean annual soil water 18O at each latitude for all terrestrial longitudes, and the lower and upper dashed lines represent the winter and summer means at each latitude, respectively. The error bars represent uncertainty.
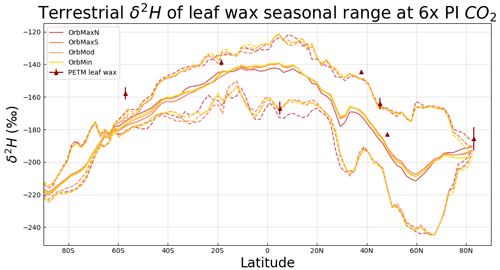
Figure A10The seasonal range of model-inferred leaf wax 2H for each orbit under 6 × PI CO2 conditions compared to PETM leaf wax 2H records. The middle solid lines represent the mean annual leaf wax 2H at each latitude for all terrestrial longitudes, and the lower and upper dashed lines represent the winter and summer means at each latitude, respectively. The error bars represent uncertainty.
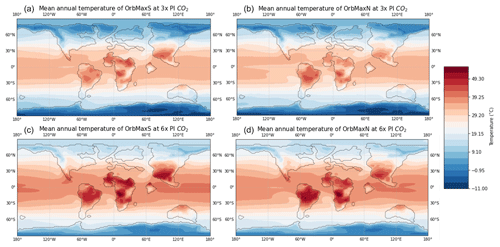
Figure A11The mean annual surface air temperature at 3 × PI CO2 (a, b) and 6 × PI CO2 (c, d) for OrbMaxS (a, c) and OrbMaxN (b, d).
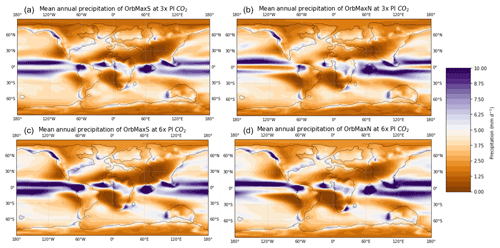
Figure A12The mean annual precipitation at 3 × PI CO2 (a, b) and 6 × PI CO2 (c, d) for OrbMaxS (a, c) and OrbMaxN (b, d). The maximum positive precipitation difference on the corresponding color bar represents anything experiencing 10 mm d−1 or higher.
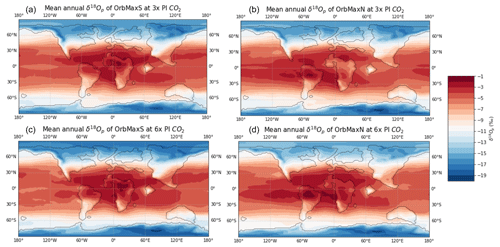
Figure A13The mean annual 18Op at 3 × PI CO2 (a, b) and 6 × PI CO2 (c, d) for OrbMaxS (a, c) and OrbMaxN (b, d).
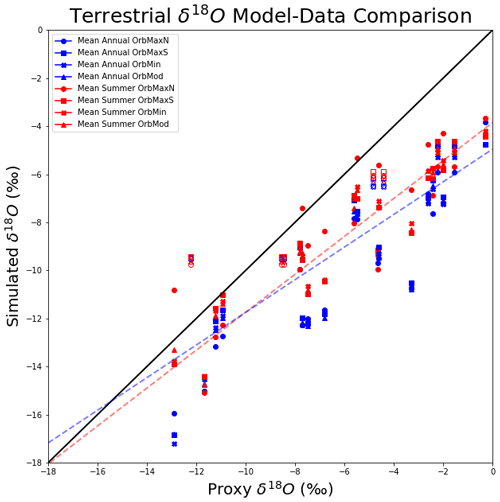
Figure A14The mean annual and mean summer soil water 18O at 6 × PI CO2 for all four orbital simulations compared to the proxy record 18O. Orbit is denoted by marker shape, time of year is denoted by marker color, unfilled markers represent paleosol carbonate records, and filled markers represent siderite records. The simulated 18O is taken from the approximate location in the model that corresponds to the location of each given proxy record. The dotted lines represent trend lines for the mean annual and mean summer values. The mean annual slope (blue) is 0.679 and the mean summer slope (red) is 0.789, suggesting that simulated mean summer isotopic signals are in closer alignment to the proxy data.
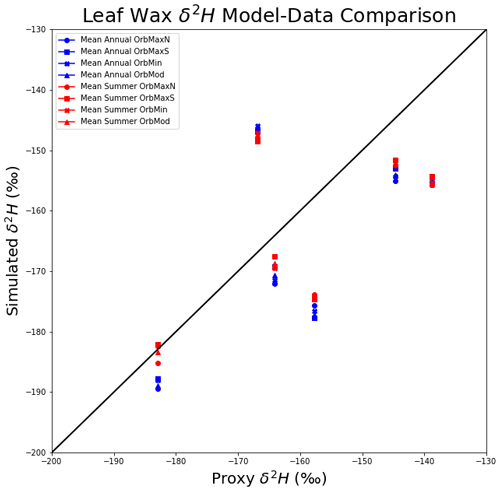
Figure A15The mean annual and mean summer model-inferred leaf wax 2H at 6 × PI CO2 for all four orbital simulations compared to the leaf wax record 2H. Orbit is denoted by marker shape and time of year is denoted by marker color. The simulated 2H is taken from the approximate location in the model that corresponds to the location of each given proxy record.
All data needed to evaluate the conclusions in the paper are presented in the paper. The published proxy records used in this study are all cited. Additional data related to this paper are available in the Zenodo repository (https://doi.org/10.5281/zenodo.7971738, Campbell, 2023). Additional data can be provided upon request to the authors. The CESM model code is available through the National Center for Atmospheric Research software development repository (https://svn-ccsm-models.cgd.ucar.edu/cesm1/exp_tags/pcesm_cesm1_2_2_tags/dt-cesm1.0_cesm1_2_2_1/, National Center for Atmospheric Research, 2019).
JC and CP designed the experimental approach. JC analyzed the results and prepared the figures. JZ developed the model code and performed the simulations. JT provided proxy data. JK provided code templates for analysis. JC prepared the manuscript, and all co-authors provided comments.
The contact author has declared that none of the authors has any competing interests.
Publisher's note: Copernicus Publications remains neutral with regard to jurisdictional claims made in the text, published maps, institutional affiliations, or any other geographical representation in this paper. While Copernicus Publications makes every effort to include appropriate place names, the final responsibility lies with the authors.
We would like to thank the editor Yannick Donnadieu, as well as the reviewers, Gordon Inglis and an anonymous reviewer, for their help in improving the manuscript.
This research has been supported by the National Science Foundation, Directorate for Geosciences (grant no. 2002397).
This paper was edited by Yannick Donnadieu and reviewed by two anonymous referees.
Anagnostou, E., John, E., Babila, T., Sexton, P., Ridgwell, A., Lunt, D., Pearson, P., Chalk, T., Pancost, R., and Foster, G.: Proxy evidence for state-dependence of climate sensitivity in the Eocene greenhouse, Nat. Commun., 11, 4436, https://doi.org/10.1038/s41467-020-17887-x, 2020.
Bartlein, P. J. and Shafer, S. L.: Paleo calendar-effect adjustments in time-slice and transient climate-model simulations (PaleoCalAdjust v1.0): impact and strategies for data analysis, Geosci. Model Dev., 12, 3889–3913, https://doi.org/10.5194/gmd-12-3889-2019, 2019.
Bataille, C., Watford, D., Ruegg, S., Lowe, A., and Bowen, G.: Chemostratigraphic age model for the Tornillo Group: A possible link between fluvial stratigraphy and climate, Palaeogeogr. Palaeocl., 457, 277–289, https://doi.org/10.1016/j.palaeo.2016.06.023, 2016.
Berger, A.: Milankovitch theory and climate, Rev. Geophys., 26, 624–657, https://doi.org/10.1029/RG026i004p00624, 1988.
Bowen, G., Maibauer, B., Kraus, M., Rohl, U., Westerhold, T., Steimke, A., Gingerich, P., Wing, S., and Clyde, W.: Two massive, rapid released of carbon during the onset of the Paleocene–Eocene thermal maximum, Nat. Geosci., 8, 44–47, https://doi.org/10.1038/ngeo2316, 2014.
Brady, E., Stevenson, S., Bailey, D., Liu, Z., Noone, D., Nusbaumer, J., Otto-Bliesner, B., Tabor, C., Tomas, R., Wong, T., Zhang, J., and Zhu, J.: The connected isotopic water cycle in the community earth system model version 1, J. Adv. Model Earth Sy., 11, 2547–2566, https://doi.org/10.1029/2019MS001663, 2019.
Burgener, L., Huntington, K., Hoke, G., Schauer, A., Ringham, M., Latorre, C., and Diaz, F.: Variations in soil carbonate formation and seasonal bias over > 4 km of relief in the western Andes revealed by clumped isotope thermometry, Earth Planet Sc. Lett., 441, 188–199, https://doi.org/10.1016/j.epsl.2016.02.033, 2016.
Byrne, M. and O'Gorman, P.: Land-ocean warming contrast over a wide range of climates: Convective quasi-equilibrium theory and idealized simulations, J. Climate, 26, 4000–4016, https://doi.org/10.1175/jcli-d-12-00262.1, 2013.
Byrne, M. and Schneider, T.: Narrowing of the ITCZ in a warming climate: Physical mechanisms, Geophys. Res. Lett., 43, 350–357, https://doi.org/10.1002/2016GL070396, 2016.
Campbell, J.: CESM1.2 simulation data for “CO2 and orbitally driven oxygen isotope variability in the Early Eocene”, Zenodo [data set], https://doi.org/10.5281/zenodo.7971738, 2023.
Craig, H.: Isotopic variations in meteoric waters, Science, 133, 1702–1703, https://doi.org/10.1126/science.133.3465.1702, 1961.
Cramwinckel, M., Burls, N., Fahad, A., Knapp, S., West, C., Reichgelt, T., Greenwood, D., Chan, W., Donnadieu, Y., Hutchinson, D., de Boer, A., Ladant, J., Morozova, P., Niezgodzki, I., Knorr, G., and Inglis, G.: Global and zonal-mean hydrological response to early Eocene warmth, Palaeoceanography, 38, 6, https://doi.org/10.1029/2022PA004542, 2023.
Dai, J., Zhang, X., Luo, Z., Liu, Z., He, X., Rao, Z., Guan, H.: Variation of the stable isotopes of water in the soil-plant-atmosphere continuum of a Cinnamomum camphora woodland in the East Asian monsoon region, J. Hydrol., 589, https://doi.org/10.1016/j.jhydrol.2020.125199, 2020.
Dansgaard, W.: Stable isotopes in precipitation, Tellus, 16, 436–468, https://doi.org/10.1111/j.2153-3490.1964.tb00181.x, 1964.
Davis, B. and Brewer, S.: A unified approach to orbital, solar, and lunar forcing based on the earth's latitudinal insolation/temperature gradient, Quaternary Sci. Rev., 30, 1861–1874, https://doi.org/10.1016/j.quascirev.2011.04.016, 2011.
Dong, B., Gregory, J., and Sutton, R.: Understanding land–sea warming contrast in response to increasing greenhouse gases, Part I: Transient adjustment, J. Climate, 22, 3079–3097, https://doi.org/10.1175/2009JCLI2652.1, 2009.
Douville, H., Raghavan, K., and Renwick, J.: Water cycle changes, in: Climate Change 2021: The Physical Science Basis. Contribution of working group I to the sixth assessment report of the Intergovernmental Panel on Climate Change, Cambridge University Press, 1055–1210, https://doi.org/10.1017/9781009157896.010, 2021.
Fernandez, A., Tang, J., and Rosenheim, B.: Siderite `clumped' isotope thermometry: A new paleoclimate proxy for humid continental environments, Geochim. Cosmochim. Ac., 126, 411–421, https://doi.org/10.1016/j.gca.2013.11.006, 2014.
Flato, G. and Marotzke, J.: Evaluation of climate models, in: Climate Change 2013: The Physical Science Basis. Contribution of working group I to the fifth assessment report of the Intergovernmental Panel on Climate Change, Cambridge University Press, 741–866, https://doi.org/10.1017/CBO9781107415324, 2013.
Friedman, I. and O'Neil, J.: Compilation of stable isotope fractionation factors of geochemical interest, in: Data of Geochemistry, USGS, https://doi.org/10.3133/pp440KK, 1977.
Galeotti, S., Krishnan, S., Pagani, M., Lanci, L., Gaudio, A., Zachos, J., Monechi, S., Morelli, G., and Lourens, L.: Orbital chronology of early Eocene hyperthermals from the Contessa Road section, central Italy, Earth Planet Sc. Lett., 290, 192–200, 192–200. https://doi.org/10.1016/j.epsl.2009.12.021, 2010.
Garel, S., Schnyder, J., Jacob, J., Dupuis, C., Boussafir, M., Milbeau, C., Storme, J-Y., Iakovleva, A., Yans, J., Baudin, F., Flehoc, C., and Quesnel, F.: Paleohydrological and paleoenvironmental changes recorded in terrestrial sediments of the Paleocene–Eocene boundary (Normandy, France), Palaeogeogr. Palaeocl., 376, 184–199, https://doi.org/10.1016/j.palaeo.2013.02.035, 2013.
Gat, J. R.: Atmospheric water balance – the isotopic perspective, Hydrol. Process., 14, 1357–1369, https://doi.org/10.1002/1099-1085(20000615)14:8<1357::AID-HYP986>3.0.CO;2-7, 2000.
Gat, J. R. and Airey, P. L.: Stable water isotopes in the atmosphere/biosphere/lithosphere interface: Scaling-up from the local to continental scale, under humid and dry conditions, Global Planet. Change, 51, 25–33, https://doi.org/10.1016/j.gloplacha.2005.12.004, 2006.
Gierz, P., Werner, M., and Lohmann, G.: Simulating climate and stable water isotopes during the Last Interglacial using a coupled climate-isotope model, J. Adv. Model. Earth Sy., 9, 2027–2045, https://doi.org/10.1002/2017MS001056, 2017.
Handley, L., Pearson, P., McMillan, I., and Pancost, R.: Large terrestrial and marine carbon and hydrogen isotope excursions in a new Paleocene/Eocene boundary section from Tanzania, Earth Planet Sc. Lett., 275, 17–25, https://doi.org/10.1016/j.epsl.2008.07.030, 2008.
Handley, L., Crouch, E., and Pancost, R.: A New Zealand record of sea level rise and environmental change during the Paleocene–Eocene thermal maximum, Palaeogeogr. Palaeocl., 305, 185–200, https://doi.org/10.1016/j.palaeo.2011.03.001, 2011.
Herold, N., Buzan, J., Seton, M., Goldner, A., Green, J. A. M., Müller, R. D., Markwick, P., and Huber, M.: A suite of early Eocene ( 55 Ma) climate model boundary conditions, Geosci. Model Dev., 7, 2077–2090, https://doi.org/10.5194/gmd-7-2077-2014, 2014.
Huber, M. and Caballero, R.: The early Eocene equable climate problem revisited, Clim. Past, 7, 603–633, https://doi.org/10.5194/cp-7-603-2011, 2011.
Hurrell, J., Holland, M., Gent, P., Ghan, S., Kay, J., Kushner, P., Lamarque J., Large, W., Lawrence, D., Lindsay, K., Lipscomb, W., Long, M., Mahowald, N., Marsh, D., Neale, R., Rasch, R., Vavrus, S., Vertenstein, M., Bader, D., Collins, W., Hack, J., Kiehl, J., and Marshall, S.: The community earth system model: A framework for collaborative research, B. Am. Meteorol. Soc., 94, 1339–1360, https://doi.org/10.1175/bams-d-12-00121.1, 2013.
Inglis, G., Bhattacharya, T., Hemingway, J., Hollingsworth, E., Feakins, S., and Tierney, J.: Biomarker approaches for reconstructing terrestrial environmental change, Annu. Rev. Earth Pl. Sc., 50, 369–394, https://doi.org/10.1146/annurev-earth-032320-095943, 2022.
Inglis, G. N., Bragg, F., Burls, N. J., Cramwinckel, M. J., Evans, D., Foster, G. L., Huber, M., Lunt, D. J., Siler, N., Steinig, S., Tierney, J. E., Wilkinson, R., Anagnostou, E., de Boer, A. M., Dunkley Jones, T., Edgar, K. M., Hollis, C. J., Hutchinson, D. K., and Pancost, R. D.: Global mean surface temperature and climate sensitivity of the early Eocene Climatic Optimum (EECO), Paleocene–Eocene Thermal Maximum (PETM), and latest Paleocene, Clim. Past, 16, 1953–1968, https://doi.org/10.5194/cp-16-1953-2020, 2020.
Jaramillo, C., Ochoa, D., Contreras, L., Pagani, M., Carvajal-Ortiz, H., Pratt, L., Krishnan, S., Cardonna, A., Romero, M., Quiroz, L., Rodriguez, G., Rueda, M., de la Parra, F., Moron, S., Green, W., Bayona, G., Montes, C., Quintero, O., Ramirez, R., Mora, G., Schouten, S., Bermudez, H., Navarrete, R., Parra, F., Alvaran, M., Osorno, J., Crowley, J., Valencia, V., and Vervoort, J.: Effects of rapid global warming at the Paleocene–Eocene boundary on neotropical vegetation, Science, 330, 957–961, https://doi.org/10.1126/science.1193833, 2010.
Kahmen, A., Hoffmann, B., Schefub, E., Arndt, S., Cernusak, L., West, J., and Sachse, D.: Leaf water deuterium enrichment shapes leaf wax n-alkane ??D values of angiosperm plants II: Observational evidence and global implications, Geochim. Cosmochim. Ac., 111, 50–63, https://doi.org/10.1016/j.gca.2012.09.004, 2013.
Keery, J. S., Holden, P. B., and Edwards, N. R.: Sensitivity of the Eocene climate to CO2 and orbital variability, Clim. Past, 14, 215–238, https://doi.org/10.5194/cp-14-215-2018, 2018.
Kelson, J., Watford, D., Bataille, C., Huntington, K., Hyland, E., and Bowen, G.: Warm terrestrial subtropics during the Paleocene and Eocene: Carbonate clumped isotope evidence from the Tornillo Basin, Texas (USA), Palaeogeogr. Palaeocl. 33, 1230–1249, https://doi.org/10.1029/2018pa003391, 2018.
Kelson, J., Huntington, K., Breecker, D., Burgener, L., Gallagher, T., Hoke, G., and Petersen, S.: A proxy for all seasons? A synthesis of clumped isotope data from Holocene soil carbonates, Quaternary Sci. Rev., 234, https://doi.org/10.1016/j.quascirev.2020.106259, 2020.
Kiehl, J., Shields, C., Snyder, M., Zachos, J., and Rothstein, M.: Greenhouse and orbital forced climate extreme during the early Eocene, Philos. T. R. Soc. A, 376, 2130, https://doi.org/10.1098/rsta.2017.0085, 2018.
Koch, P., Zachos, J., and Dettman, D.: Stable isotope stratigraphy and paleoclimatology of the Paleogene Bighorn Basin (Wyoming, USA), Palaeogeogr. Palaeocl., 115, 61–89, https://doi.org/10.1016/0031-0182(94)00107-j, 1995.
Konecky, B., Dee, S., and Noone, D.: WaxPSM: A forward model of leaf wax hydrogen isotope ratios to bridge proxy and model estimates of past climate, J. Geophys. Res.-Biogeo., 124, 2107–2125, https://doi.org/10.1029/2018JG004708, 2019a.
Konecky, B., Noone, D., and Cobb, K.: The influence of competing hydroclimate processes on stable isotope ratios in tropical rainfall, Geophys. Res. Lett., 46, 1622–1633, https://doi.org/10.1029/2018GL080188, 2019b.
Lawrence, K., Sloan, L., and Sewall, J.: Terrestrial climatic response to precessional orbital forcing in the Eocene, in: Causes and Consequences of Globally Warm Climates in the Early Paleogene, Geol. Soc. Am., 369, https://doi.org/10.1130/0-8137-2369-8.65, 2003.
Lourens, L., Sluijs, A., Zachos, J., Thomas, E., Rohl, U., Bowles, J., and Raffi, I.: Astronomical pacing of late Paleocene to early Eocene global warming events, Nature, 435, 1083–1087, https://doi.org/10.1038/nature03814, 2005.
Lunt, D., Ridgwell, A., Sluijs, A., Zachos, J., Hunter, S., and Haywood, A.: A model for orbital pacing of methane hydrate destabilization during the Paleogene, Nat. Geosci., 4, 775–778, https://doi.org/10.1038/ngeo1266, 2011.
Luz, B., Barkan, E., Yam, R., and Shemesh, A.: Fractionation of oxygen and hydrogen isotopes in evaporating water, Geochim. Cosmochim. Ac., 73, 6697–6703, https://doi.org/10.1016/j.gca.2009.08.008, 2009.
Mulch, A., Teyssier, C., Cosca, M., and Chamberlain, C.: Stable isotope paleoaltimetry of Eocene core complexes in the North American Cordillera, Tectonics, 26, 4, https://doi.org/10.1029/2006TC001995, 2007.
Muller, R., Cannon, J., Qin, X., Watson, R., Gurnis, M., Williams, S., Pfaffelmoser, T., Seton, M., Russell, S., and Zahirovic, S.: GPlates: Building a virtual earth through deep time, Geochem. Geophy. Geosy., 19, 2243–2261, https://doi.org/10.1029/2018GC007584, 2018.
National Center for Atmospheric Research: The Community Earth System Model version 1.2.2, SVN [code], https://svn-ccsm-models.cgd.ucar.edu/cesm1/exp_tags/pcesm_cesm1_2_2_tags/dt-cesm1.0_cesm1_2_2_1/ (last access: 11 March 2024), 2019.
Pagani, M., Pedentchouk, N., Huber, M., Sluijs, A., Schouten, S., Brinkhuis, H., Damste, J., Dickens, G., and Expedition 302 Scientists: Arctic hydrology during global warming at the Paleocene/Eocene thermal maximum, Nature, 442, 671–675, https://doi.org/10.1038/nature05043, 2006.
Piedrahita, V., Galeotti, S., Zhao, X., Roberts, A., Rohling, E., Heslop, D., Florindo, F., Grant, K., Rodríguez-Sanz, L., Reghellin, D., and Zeebe, R.: Orbital phasing of the Paleocene–Eocene Thermal Maximum, Earth Planet Sc. Lett., 598, 117839, https://doi.org/10.1016/j.epsl.2022.117839, 2022.
Poulsen, C. and Jeffery, M.: Climate change imprinting on stable isotopic compositions of high-elevation meteoric water cloaks past surface elevations of major orogens, Geology, 39, 595–598, https://doi.org/10.1130/g32052.1, 2011.
Poulsen, C., Pollard, D., and White, T.: General circulation model simulation of the δ18O content of continental precipitation in the middle Cretaceous: A model-proxy comparison, Geology, 35, 199–202, https://doi.org/10.1130/g23343a.1, 2007.
Rae, J., Zhang, Y., Liu, X., Foster, G., Stoll, H., and Whiteford, R.: Atmospheric CO2 over the past 66 million years from marine archives, Annu. Rev. Earth Pl. Sc., 49, 609–641, https://doi.org/10.1146/annurev-earth-082420-063026, 2021.
Risi, C., Galewsky, J., Reverdin, G., and Brient, F.: Controls on the water vapor isotopic composition near the surface of tropical oceans and role of boundary layer mixing processes, Atmos. Chem. Phys., 19, 12235–12260, https://doi.org/10.5194/acp-19-12235-2019, 2019.
Sachse, D., Billault, I., Bowen, G., Chikaraishi, Y., Dawson, T., Feakins, S., Freeman, K., Magill, C., McInerney, F., van der Meer, M., Polissar, P., Robins, R., Sachs, J., Schmidt, H-L., Sessions, A., White, J., West, J., and Kahmen, A.: Molecular paleohydrology: Interpreting the hydrogen-isotopic composition of lipid biomarkers from photosynthesizing organisms, Annu. Rev. Earth Pl. Sc., 40, 221–249, https://doi.org/10.1146/annurev-earth-042711-105535, 2012.
Singh, H., Bitz, C., Donohoe, A., Nusbaumer, J., and Noone, D.: A mathematical framework for analysis of water tracers. Part II: Understanding large-scale perturbations in the hydrological cycle dur to CO2 doubling, J. Climate, 29, 6765–6782, https://doi.org/10.1175/JCLI-D-16-0293.1, 2016.
Smith, F., Wing, S., and Freeman, K.: Magnitude of the carbon isotope excursion at the Paleocene–Eocene thermal maximum: The role of plant community change, Earth Planet Sc. Lett., 262, 50–65, https://doi.org/10.1016/j.epsl.2007.07.021, 2007.
Snell, K., Thrasher, B., Eiler, J., Koch, P., Sloan, L., and Tabor, N.: Hot summers in the Bighorn Basin during the early Paleogene, Geology, 41, 55–58, https://doi.org/10.1130/g33567.1, 2013.
Tardif, D., Toumoulin, A., Frédéric, F., Donnadieu, Y., Le Hir, G., Barbolini, N., Licht, A., Ladant, J. B., Sepulchre, P., Viovy, N., Hoorn, C., and Dupont-Nivet, G.: Orbital variations as a major driver of climate and biome distribution during the greenhouse to icehouse transition, Sci. Adv., 7, 43, https://doi.org/10.1126/sciadv.abh2819, 2021.
Thompson, A., Zhu, J., Poulsen, C., Tierney, J., and Skinner, C.: Northern Hemisphere vegetation change drives a Holocene thermal maximum, Science, 8, 15, https://doi.org/10.1126/sciadv.abj6535, 2022.
Tichy, H., Hellwisg, M., and Kallina, W.: Revisiting theories of humidity transduction: A focus on electrophysiological data, Front. Physiol., 8, 1, https://doi.org/10.3389/fphys.2017.00650, 2017.
Tierney, J., Poulsen, C., Montanez, I., Bhattacharya, T., Feng, R., Ford, H., Honisch, B., Inglis, G., Petersen, S., Sagoo, N., Tabor, C., Thirumalai, K., Zhu, J., Burls, N., Foster, G., Godderis, Y., Huber, B., Ivany, L., Turner, S., Lunt, D., Mcelwain, J., Mills, B., Otto-Bliesner, B., Ridgwell, A., and Zhang, Y.: Past climates inform our future, Science, 370, 6517, https://doi.org/10.1126/science.aay3701, 2020.
Tierney, J., Zhu, J., Li, M., Ridgwell, A., Hakim, G., Poulsen, C., Whiteford, R., Raw, J., and Kump, L.: Spatial patterns of climate change across the Paleocene–Eocene thermal maximum, P. Natl. Acad. Sci. USA, 119, e2205326119, https://doi.org/10.1073/pnas.2205326119, 2022.
Tipple, B., Pagani, M., Krishnan, S., Dirghangi, S., Galeotti, S., Agnini, C., Giusberti, L., and Rio D.: Coupled high-resolution marine and terrestrial records of carbon and hydrologic cycles variations during the Paleocene–Eocene thermal maximum (PETM), Earth Planet Sc. Lett., 311, 82–92, https://doi.org/10.1016/j.epsl.2011.08.045, 2011.
Westerhold, T., Rohl, U., McCarren, H., and Zachos, J.: Latest on the absolute age of the Paleocene–Eocene thermal maximum (PETM): New insights from exact stratigraphic position of key ash layers +19 and −17, Earth Planet Sc. Lett., 287, 412–419, https://doi.org/10.1016/j.epsl.2009.08.027, 2009.
Westerhold, T., Rohl, U., and Laskar, J.: Time scale controversy: Accurate orbital calibration of the early Paleogene, Geochem. Geophy. Geosy., 13, Q06015, https://doi.org/10.1029/2012gc004096, 2012.
White, T., Bradley, D., Haeussler, P., and Rowley, D.: Late Paleocene-early Eocene paleosols and a new measure of the transport distance of Alaska's Yakutat terrane, J. Geol., 125, 113–123, https://doi.org/10.1086/690198, 2017.
van Dijk, J., Fernandez, A., Muller, I., Lever, M., and Bernasconi S.: Oxygen isotope fractionation in the siderite-water system between 8.5 and 62 °C, Geochim. Cosmochim. Ac., 220, 535–551, https://doi.org/10.1016/j.gca.2017.10.009, 2018.
van Dijk, J., Fernandez, A., Storch, J., White, T., Lever, M., Muller, I., Bishop, S., Seifert, R., Driese, S., Krylov, A., Ludvigson, G., Turchyn, A., Link, C., Wittkop, C., and Bernasconi, S.: Experimental calibration of clumped isotopes in siderite between 8.5 and 62 °C and its application as paleo-thermometer in paleosols, Geochim. Cosmochim. Ac., 254, 1–20, https://doi.org/10.1016/j.gca.2019.03.018, 2019.
van Dijk, J., Fernandez, A., Bernasconi, S., Rugenstein, J., Passey, S., and White, T.: Spatial pattern of super-greenhouse warmth controlled by elevated specific humidity, Nat. Geosci., 13, 739–744, https://doi.org/10.1038/s41561-020-00648-2, 2020.
Zachos, J., McCarren, H., Murphy, B., Rohl, U., and Westerhold, T.: Tempo and scale of late Paleocene and early Eocene carbon isotope cycles: Implications for the origin of hyperthermals, Earth Planet. Sc. Lett., 299, 242–249, https://doi.org/10.1016/j.epsl.2010.09.004, 2010.
Zeebe, R., Westerhold, T., Littler, K., and Zachos, J.: Orbital forcing of the Paleocene and Eocene carbon cycle, Palaeoceanography, 32, 440–465, https://doi.org/10.1002/2016pa003054, 2017.
Zhu, J., Liu, Z., Otto-Bliesner, B., Brady, E., Noone, D., Zhang, J., Tomas, R., Jahn, A., Nusbaumer, J., and Wong, T.: Reduced ENSO variability at the LGM revealed by an isotope-enabled earth system model, Geophys. Res. Lett., 44, 6984–6992, https://doi.org/10.1002/2017GL073406, 2017.
Zhu, J., Poulsen, C., and Tierney, J.: Simulation of Eocene extreme warmth and high climate sensitivity through cloud feedbacks, Science, 5, eaax1874, https://doi.org/10.1126/sciadv.aax1874, 2019.
Zhu, J., Poulsen, C., Otto-Bliesner, B., Liu, Z., Brady, E., and Noone, D.: Simulation of Early Eocene water isotopes using an Earth system model and its implication for past climate reconstruction, Earth Planet Sc. Lett., 537, https://doi.org/10.1016/j.epsl.2020.116164, 2020.